Post History
#3: Post edited
Alas, it isn't clear to me that** it becomes clear that the- probabilities are not 50-50 for the two unopened doors**. Had I never seen this exercise or problem, even if there were 1 **B**illion doors, I would "**stubbornly stick with their original choice**".
- What am I misunderstanding? Am I just that witless? Rocketing the number of doors hasn't assisted me to intuit the Monty Hall Problem.
- >To build correct intuition, let's consider an extreme case. Suppose that there are
- a million doors, 999,999 of which contain goats and 1 of which has a car. After
- the contestant's initial pick, Monty opens 999,998 doors with goats behind them
- and others the choice to switch. In this extreme case, **it becomes clear that the
- probabilities are not 50-50 for the two unopened doors; very few people would
- stubbornly stick with their original choice**. The same is true for the three-door case.
- Blitzstein. *Introduction to Probability* (2019 2 ed). p 71.
- [The Time Everyone “Corrected” the World’s Smartest Woman](https://priceonomics.com/the-time-everyone-corrected-the-worlds-smartest/)
- > Here’s another way to visualize this. Imagine that instead of three doors, Monty Hall presents you with 100 doors; behind 99 of them are goats, and behind one of them is the car. You select door #1, and your initial odds of winning the car are now 1/100:
- >
- > 
- >
- > Then, let’s suppose that Monty Hall opens 98 of the other doors, revealing a goat behind each one. Now you’re left with two choices: keep door #1, or switch to door #100:
- >
- > 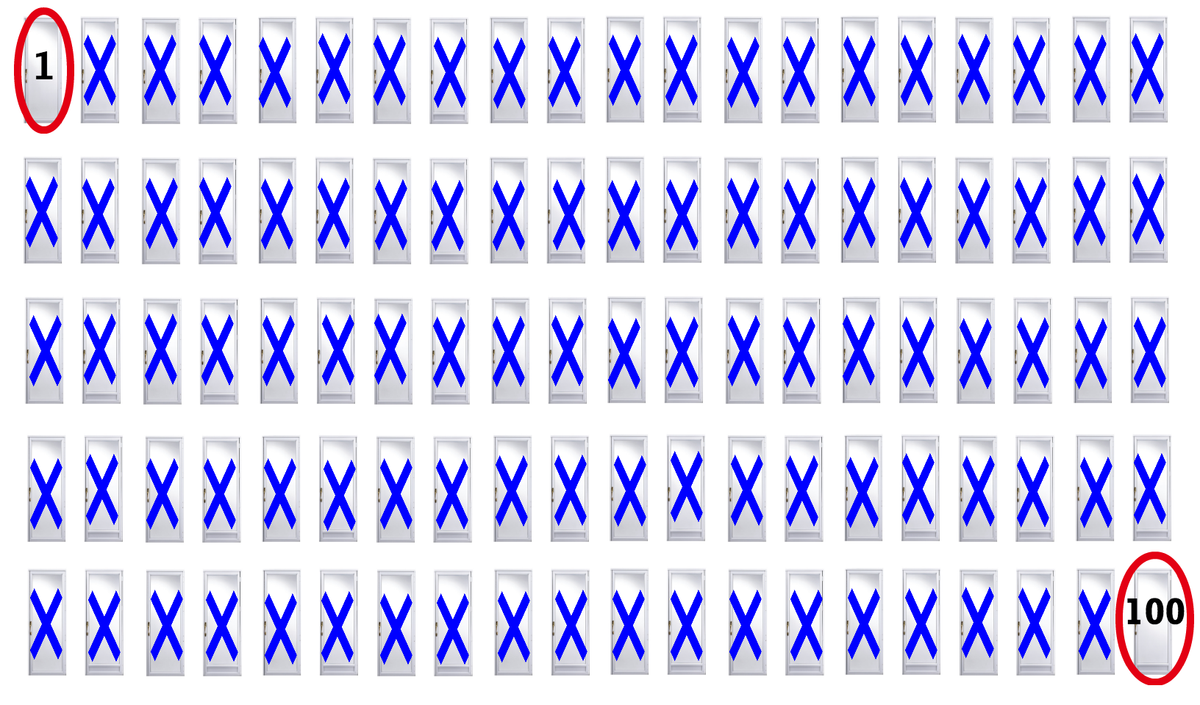
- >
- > When you select door #1, there is a 99/100 chance that the car is behind one of the other doors. The fact that Monty Hall reveals 98 goats does not change these initial odds -- it merely "shifts" that 99/100 chance to door #100. You can either stick with your original 1/100 odds pick, or switch to door #100, with a much higher probability of winning the car.
- Alas, it isn't clear to me that **it becomes clear that the
- probabilities are not 50-50 for the two unopened doors**. Had I never seen this exercise or problem, even if there were 1 **B**illion doors, I would "**stubbornly stick with their original choice**".
- What am I misunderstanding? Am I just that witless? Rocketing the number of doors hasn't assisted me to intuit the Monty Hall Problem.
- >To build correct intuition, let's consider an extreme case. Suppose that there are
- a million doors, 999,999 of which contain goats and 1 of which has a car. After
- the contestant's initial pick, Monty opens 999,998 doors with goats behind them
- and others the choice to switch. In this extreme case, **it becomes clear that the
- probabilities are not 50-50 for the two unopened doors; very few people would
- stubbornly stick with their original choice**. The same is true for the three-door case.
- Blitzstein. *Introduction to Probability* (2019 2 ed). p 71.
- [The Time Everyone “Corrected” the World’s Smartest Woman](https://priceonomics.com/the-time-everyone-corrected-the-worlds-smartest/)
- > Here’s another way to visualize this. Imagine that instead of three doors, Monty Hall presents you with 100 doors; behind 99 of them are goats, and behind one of them is the car. You select door #1, and your initial odds of winning the car are now 1/100:
- >
- > 
- >
- > Then, let’s suppose that Monty Hall opens 98 of the other doors, revealing a goat behind each one. Now you’re left with two choices: keep door #1, or switch to door #100:
- >
- > 
- >
- > When you select door #1, there is a 99/100 chance that the car is behind one of the other doors. The fact that Monty Hall reveals 98 goats does not change these initial odds -- it merely "shifts" that 99/100 chance to door #100. You can either stick with your original 1/100 odds pick, or switch to door #100, with a much higher probability of winning the car.
#2: Post edited
- Alas, it isn't clear to me that** it becomes clear that the
- probabilities are not 50-50 for the two unopened doors**. Had I never seen this exercise or problem, even if there were 1 **B**illion doors, I would "**stubbornly stick with their original choice**".
- What am I misunderstanding? Am I just that witless? Rocketing the number of doors hasn't assisted me to intuit the Monty Hall Problem.
- >To build correct intuition, let's consider an extreme case. Suppose that there are
- a million doors, 999,999 of which contain goats and 1 of which has a car. After
- the contestant's initial pick, Monty opens 999,998 doors with goats behind them
- and others the choice to switch. In this extreme case, **it becomes clear that the
- probabilities are not 50-50 for the two unopened doors; very few people would
- stubbornly stick with their original choice**. The same is true for the three-door case.
Blitzstein. *Introduction to Probability* (2019 2 ed). p 71.
- Alas, it isn't clear to me that** it becomes clear that the
- probabilities are not 50-50 for the two unopened doors**. Had I never seen this exercise or problem, even if there were 1 **B**illion doors, I would "**stubbornly stick with their original choice**".
- What am I misunderstanding? Am I just that witless? Rocketing the number of doors hasn't assisted me to intuit the Monty Hall Problem.
- >To build correct intuition, let's consider an extreme case. Suppose that there are
- a million doors, 999,999 of which contain goats and 1 of which has a car. After
- the contestant's initial pick, Monty opens 999,998 doors with goats behind them
- and others the choice to switch. In this extreme case, **it becomes clear that the
- probabilities are not 50-50 for the two unopened doors; very few people would
- stubbornly stick with their original choice**. The same is true for the three-door case.
- Blitzstein. *Introduction to Probability* (2019 2 ed). p 71.
- [The Time Everyone “Corrected” the World’s Smartest Woman](https://priceonomics.com/the-time-everyone-corrected-the-worlds-smartest/)
- > Here’s another way to visualize this. Imagine that instead of three doors, Monty Hall presents you with 100 doors; behind 99 of them are goats, and behind one of them is the car. You select door #1, and your initial odds of winning the car are now 1/100:
- >
- > 
- >
- > Then, let’s suppose that Monty Hall opens 98 of the other doors, revealing a goat behind each one. Now you’re left with two choices: keep door #1, or switch to door #100:
- >
- > 
- >
- > When you select door #1, there is a 99/100 chance that the car is behind one of the other doors. The fact that Monty Hall reveals 98 goats does not change these initial odds -- it merely "shifts" that 99/100 chance to door #100. You can either stick with your original 1/100 odds pick, or switch to door #100, with a much higher probability of winning the car.
#1: Initial revision
Why would skyrocketing the numbers of doors help laypeople intuit the Monty Hall Problem?
Alas, it isn't clear to me that** it becomes clear that the probabilities are not 50-50 for the two unopened doors**. Had I never seen this exercise or problem, even if there were 1 **B**illion doors, I would "**stubbornly stick with their original choice**". What am I misunderstanding? Am I just that witless? Rocketing the number of doors hasn't assisted me to intuit the Monty Hall Problem. >To build correct intuition, let's consider an extreme case. Suppose that there are a million doors, 999,999 of which contain goats and 1 of which has a car. After the contestant's initial pick, Monty opens 999,998 doors with goats behind them and others the choice to switch. In this extreme case, **it becomes clear that the probabilities are not 50-50 for the two unopened doors; very few people would stubbornly stick with their original choice**. The same is true for the three-door case. Blitzstein. *Introduction to Probability* (2019 2 ed). p 71.


















