Why would skyrocketing the numbers of doors help laypeople intuit the Monty Hall Problem?
Alas, it isn't clear to me that it becomes clear that the probabilities are not 50-50 for the two unopened doors. Had I never seen this exercise or problem, even if there were 1 Billion doors, I would "stubbornly stick with their original choice".
What am I misunderstanding? Am I just that witless? Rocketing the number of doors hasn't assisted me to intuit the Monty Hall Problem.
To build correct intuition, let's consider an extreme case. Suppose that there are a million doors, 999,999 of which contain goats and 1 of which has a car. After the contestant's initial pick, Monty opens 999,998 doors with goats behind them and others the choice to switch. In this extreme case, it becomes clear that the probabilities are not 50-50 for the two unopened doors; very few people would stubbornly stick with their original choice. The same is true for the three-door case.
Blitzstein. Introduction to Probability (2019 2 ed). p 71.
The Time Everyone “Corrected” the World’s Smartest Woman
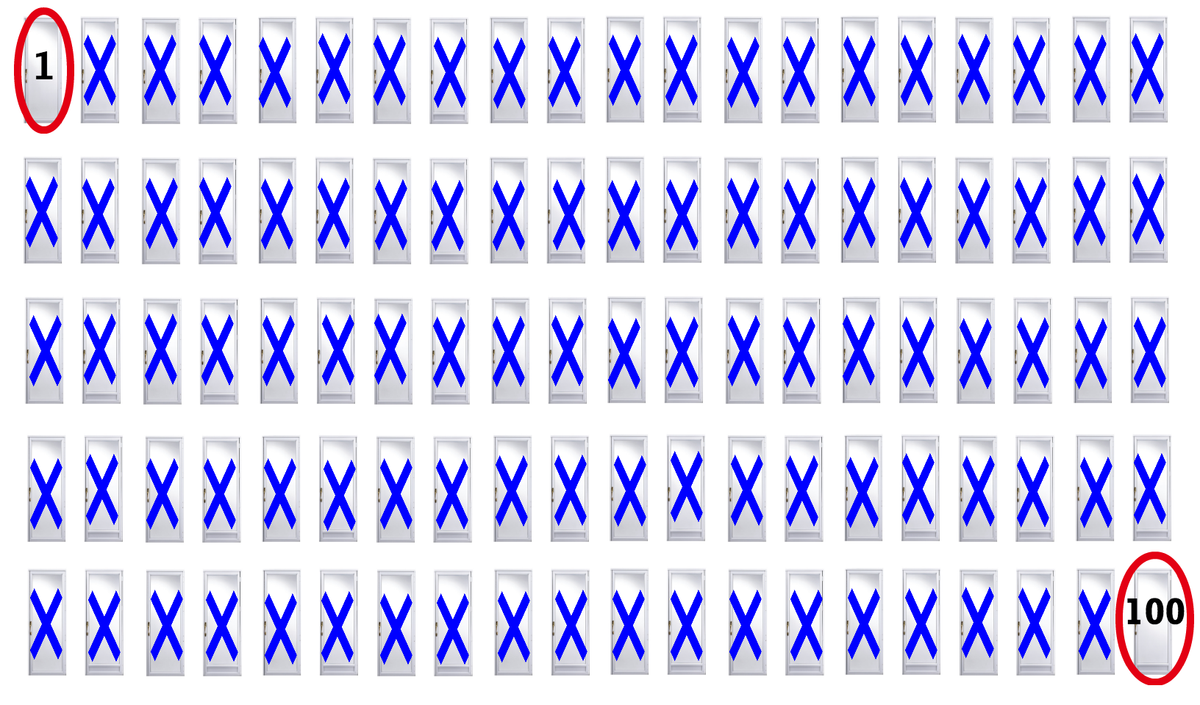
Here’s another way to visualize this. Imagine that instead of three doors, Monty Hall presents you with 100 doors; behind 99 of them are goats, and behind one of them is the car. You select door #1, and your initial odds of winning the car are now 1/100:
Then, let’s suppose that Monty Hall opens 98 of the other doors, revealing a goat behind each one. Now you’re left with two choices: keep door #1, or switch to door #100:
When you select door #1, there is a 99/100 chance that the car is behind one of the other doors. The fact that Monty Hall reveals 98 goats does not change these initial odds -- it merely "shifts" that 99/100 chance to door #100. You can either stick with your original 1/100 odds pick, or switch to door #100, with a much higher probability of winning the car.
1 answer
The only thing special about the door you chose is that you chose it, and you did so without any information, so objectively it isn't special at all. The door which the host leaves closed is special because it was chosen from the remaining doors by someone with information, so objectively it is actually special.






















0 comment threads