Post History
#4: Post edited
by
DNB
·
2021-08-05T03:41:26Z (over 3 years ago)
If a 2nd test's independent from the 1st test, then why does $\frac{0.95}{0.05}$ figure twice in $\frac{P(D|T_1)}{P(D^C|T_1)}\frac{P(T_2)|D,T_1)}{P(T_2|D^C,T_1)}$?
- If a 2nd test's independent from the 1st test, then why does $\frac{0.95}{0.05}$ figure twice in $\frac{P(D|T_1)}{P(D^C|T_1)}\frac{P(T_2|D,T_1)}{P(T_2|D^C,T_1)}$?
#3: Post edited
by
DNB
·
2021-08-05T03:40:15Z (over 3 years ago)
If a 2nd test's independent from the 1st test, then why does \frac{0.95}{0.05} figure twice in $\frac{P(D|T_1)}{P(D^C|T_1)}{P(T_2)|D,T_1)}{P(T_2|D^C,T_1)}$??
- If a 2nd test's independent from the 1st test, then why does $\frac{0.95}{0.05}$ figure twice in $\frac{P(D|T_1)}{P(D^C|T_1)}\frac{P(T_2)|D,T_1)}{P(T_2|D^C,T_1)}$?
#2: Post edited
by
DNB
·
2021-08-05T03:39:47Z (over 3 years ago)
If a 2nd test's independent from the 1st test, then why does $\frac0.950.05$ figure twice in $\frac{P(D|T_1)}{P(D^C|T_1)}{P(T_2)|D,T_1)}{P(T_2|D^C,T_1)}$??
- If a 2nd test's independent from the 1st test, then why does \frac{0.95}{0.05} figure twice in $\frac{P(D|T_1)}{P(D^C|T_1)}{P(T_2)|D,T_1)}{P(T_2|D^C,T_1)}$??
- The problem statement postulates that **"The new
test is independent of the original test (given his disease status)"**. So where did the two $\frac0.950.05$, that I underlined in red and purple, stem from?- >### Example 2.6.1 (Testing for a rare disease, continued).
- >Fred, who tested positive
- for conditionitis in Example 2.3.9, decides to get tested a second time. **The new
- test is independent of the original test (given his disease status)** and has the same
- sensitivity and specificity. Unfortunately for Fred, he tests positive a second time.
- Find the probability that Fred has the disease, given the evidence, in two ways: in
- one step, conditioning on both test results simultaneously, and in two steps, first
- updating the probabilities based on the first test result, and then updating again
- based on the second test result.
- >
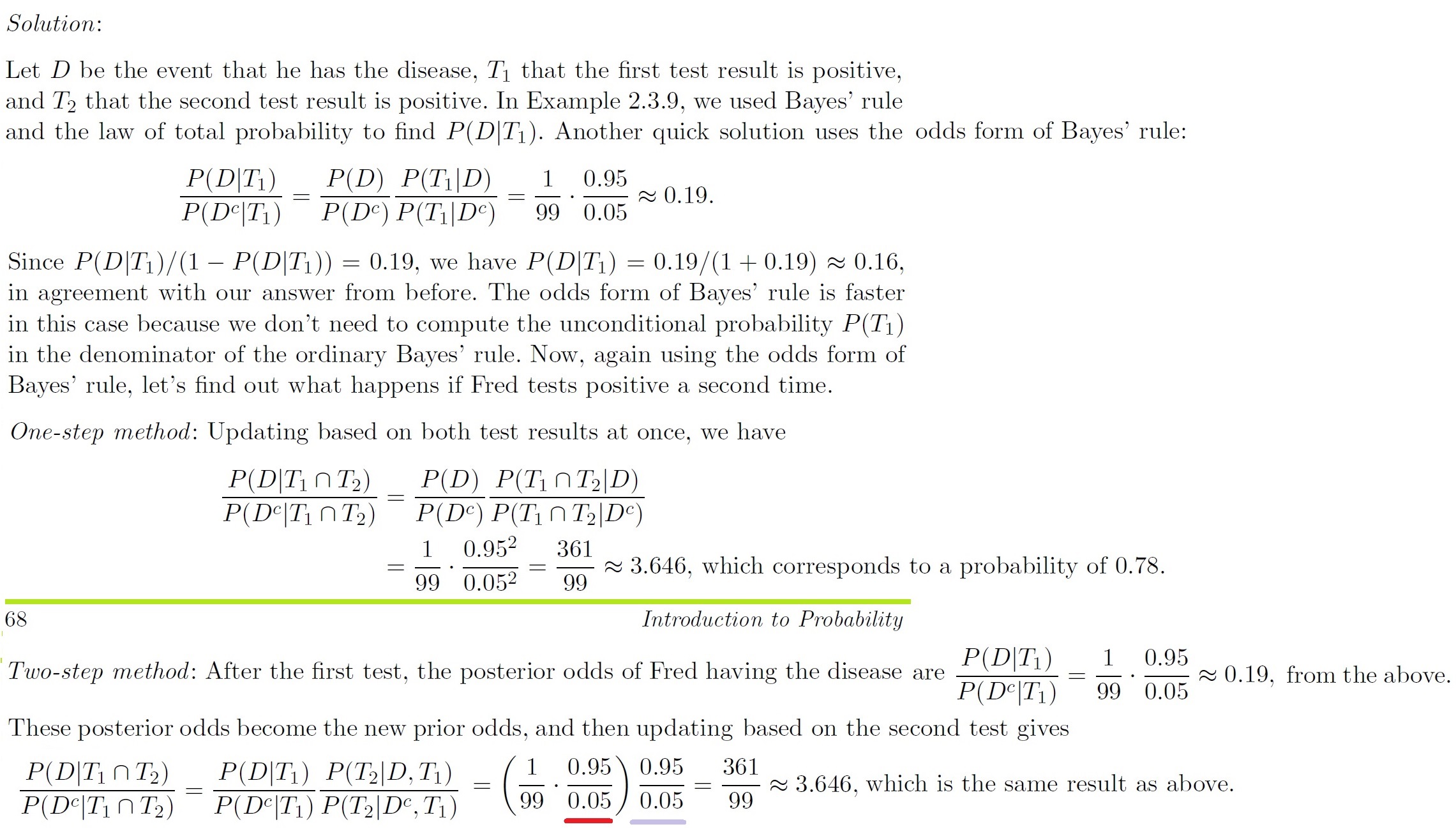
- >Note that with a second positive test result, the probability that Fred has the disease
- jumps from 0.16 to 0.78, making us much more con dent that Fred is actually
- afflicted with conditionitis. The moral of the story is that getting a second opinion
- is a good idea! □
- Blitzstein. *Introduction to Probability* (2019 2 ed). pp 67-68.
- The problem statement postulates that **"The new
- test is independent of the original test (given his disease status)"**. So where did the two $\frac{0.95}{ 0.05}$, that I underlined in red and purple, stem from?
- >### Example 2.6.1 (Testing for a rare disease, continued).
- >Fred, who tested positive
- for conditionitis in Example 2.3.9, decides to get tested a second time. **The new
- test is independent of the original test (given his disease status)** and has the same
- sensitivity and specificity. Unfortunately for Fred, he tests positive a second time.
- Find the probability that Fred has the disease, given the evidence, in two ways: in
- one step, conditioning on both test results simultaneously, and in two steps, first
- updating the probabilities based on the first test result, and then updating again
- based on the second test result.
- >
- >Note that with a second positive test result, the probability that Fred has the disease
- jumps from 0.16 to 0.78, making us much more con dent that Fred is actually
- afflicted with conditionitis. The moral of the story is that getting a second opinion
- is a good idea! □
- Blitzstein. *Introduction to Probability* (2019 2 ed). pp 67-68.
#1: Initial revision
by
DNB
·
2021-08-05T03:39:12Z (over 3 years ago)
If a 2nd test's independent from the 1st test, then why does $\frac0.950.05$ figure twice in $\frac{P(D|T_1)}{P(D^C|T_1)}{P(T_2)|D,T_1)}{P(T_2|D^C,T_1)}$??
The problem statement postulates that **"The new test is independent of the original test (given his disease status)"**. So where did the two $\frac0.950.05$, that I underlined in red and purple, stem from? >### Example 2.6.1 (Testing for a rare disease, continued). >Fred, who tested positive for conditionitis in Example 2.3.9, decides to get tested a second time. **The new test is independent of the original test (given his disease status)** and has the same sensitivity and specificity. Unfortunately for Fred, he tests positive a second time. Find the probability that Fred has the disease, given the evidence, in two ways: in one step, conditioning on both test results simultaneously, and in two steps, first updating the probabilities based on the first test result, and then updating again based on the second test result. > >Note that with a second positive test result, the probability that Fred has the disease jumps from 0.16 to 0.78, making us much more con dent that Fred is actually afflicted with conditionitis. The moral of the story is that getting a second opinion is a good idea! □ Blitzstein. *Introduction to Probability* (2019 2 ed). pp 67-68.