Mathematics

#10: Post edited by $user avatar$ DNB‭ · 2022-02-01T10:24:37Z (about 2 years ago)

Copy Link

Raw

Markdown

~~How to intuit, construe multiplicands and multiplicators $\le 10$ resulting from $\dbinom m n$ directly, WITHOUT division or factorials?~~

How to intuit, construe multiplicands and multiplicators $\le 10$ resulting from $\dbinom pc$, WITHOUT division or factorials?

I grok, am NOT asking about, the answers below. **Rather — how can I deduce and intuit the multiplicands and multiplicators $\le 10$, resulting from simplifying $\dbinom m n$ DIRECTLY? WITHOUT division or factorials!**
## Orange underline
1. Unquestionably, $\color{darkorange}{4 \times 3/2 = 3!}$ But how can I construe and intuit $\color{darkorange}{3!}$ DIRECTLY? Note that $\color{darkorange}{3!}$ wasn't one of the original numbers (2, 4) — what does $\color{darkorange}{3!}$ mean?
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write 3! explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Did I surmise correctly?
## Red underline
2. Unquestionably, $\color{red}{\dfrac{8 \times 7 \times 6}{3!} = 8 × 7}$. But how can I construe and intuit $\color{red}{8 × 7}$ DIRECTLY? Note that $\color{red}{7 } $ wasn't one of the original numbers of 3 or 8. What does $\color{red}{7 } $ mean?
**_Here's my surmisal._** You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (David Patrick, p 38 bottom), the answer ought be 8 × 7 × 6??? Why isn't there 6?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
>
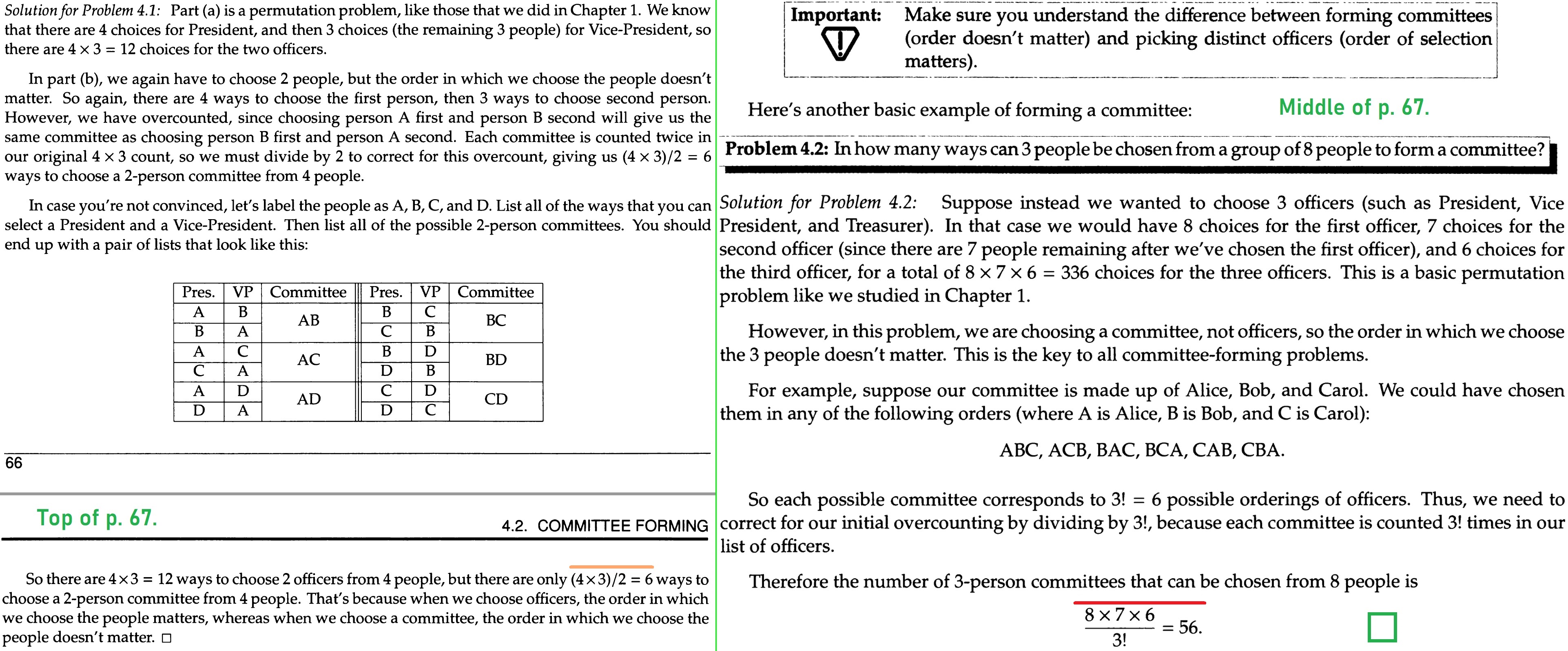
>![Image alt text](https://math.codidact.com/uploads/t7nrZLrLSsEu2HAspWbrpx4E)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.
## Examples 3 and 4
3. I know [the ways to form a committee of 2 from 8](https://mathhelpforum.com/threads/calculate-ways-to-form-a-committee-of-3-from-8-directly-without-%C3%B7.295777/post-975751) $= \dbinom8 2 = \dfrac{8\cdot7}{2!} = \color{violet}{4 × 7}.$ But how can I construe and intuit $\color{violet}{4 × 7}$ DIRECTLY? Note that $\color{violet}{4, 7}$ aren't the original numbers of 2, 8.
4. I know [the ways to form a committee of 3 from 10](https://mathforums.com/threads/calculate-ways-to-form-a-committee-of-3-from-8-directly-without-%C3%B7.362347/post-664313) $= \dbinom {10} 3 = \dfrac{10\cdot9\cdot8}{3!} = \color{springgreen}{10 × 3 × 4}.$ But how can I construe and intuit $\color{springgreen}{10 × 3 × 4}$ DIRECTLY? I'm surmising that $\color{springgreen}{10}$ refers to the original 10, and $\color{springgreen}{3}$ to the chosen 3. But what does $\color{springgreen}{4}$ mean?

I grok, am NOT asking about, the answers below. Rather — **how can I deduce and intuit the multiplicands and multiplicators $\le 10$, resulting from simplifying $\dbinom {p \text{ people}}{c\text{-person committee}}$ DIRECTLY?** ***WITHOUT division or factorials!***
## Orange underline
1. Unquestionably, $\color{darkorange}{4 \times 3/2 = 3!}$. But how can I construe, intuit $\color{darkorange}{3!}$ DIRECTLY? Note that $\color{darkorange}{3!}$ wasn't one of the original numbers (2, 4). What does $\color{darkorange}{3!}$ mean?
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write 3! explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Did I surmise correctly?
## Red underline
2. Unquestionably, $\color{red}{\dfrac{8 \times 7 \times 6}{3!} = 8 × 7}$. But how can I construe, intuit $\color{red}{8 × 7}$ DIRECTLY? Note that $\color{red}{7 } $ wasn't one of the original numbers of 3 or 8. What does $\color{red}{7 } $ mean?
**_Here's my surmisal._** You can pick the 1st committee member in $\color{red}{8} $ ways, and the 2nd member in $\color{red}{7} $ ways. Then can't you pick the 3rd member in 6 ways? By this Constructive Counting (David Patrick, p 38 bottom), the answer ought be 8 × 7 × 6? Why didn't 6 appear as a multiplicator?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
>
>![Image alt text](https://math.codidact.com/uploads/t7nrZLrLSsEu2HAspWbrpx4E)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* 2005, pp 66-7.
## Examples 3 and 4
3. I know [the ways to form a committee of 2 from 8](https://mathhelpforum.com/threads/calculate-ways-to-form-a-committee-of-3-from-8-directly-without-%C3%B7.295777/post-975751) $= \dbinom8 2 = \dfrac{8\cdot7}{2!} = \color{violet}{4 × 7}.$ But how can I construe, intuit $\color{violet}{4 × 7}$ DIRECTLY? Note that $\color{violet}{4, 7}$ aren't the original numbers of 2, 8.
4. I know [the ways to form a committee of 3 from 10](https://mathforums.com/threads/calculate-ways-to-form-a-committee-of-3-from-8-directly-without-%C3%B7.362347/post-664313) $= \dbinom {10} 3 = \dfrac{10\cdot9\cdot8}{3!} = \color{springgreen}{10 × 3 × 4}.$ But how can I construe, intuit $\color{springgreen}{10 × 3 × 4}$ DIRECTLY? I'm surmising that $\color{springgreen}{10}$ refers to the original 10, and $\color{springgreen}{3}$ to the chosen 3. But what does $\color{springgreen}{4}$ mean?

#9: Post edited by $user avatar$ DNB‭ · 2022-02-01T09:30:50Z (about 2 years ago)

Copy Link

Raw

Markdown

~~How to intuit, construe ways to form a m-member committee from p people directly, WITHOUT division or factorials?~~

How to intuit, construe multiplicands and multiplicators $\le 10$ resulting from $\dbinom m n$ directly, WITHOUT division or factorials?

I grok, am NOT asking about, the answers below. **Rather, how can I deduce and intuit the final answer DIRECTLY, WITHOUT division or factorials?**
## Orange underline
1. Unquestionably, $\color{darkorange}{4 \times 3/2 = 3!}$ But how can I construe and intuit $\color{darkorange}{3!}$ DIRECTLY? Note that $\color{darkorange}{3!}$ wasn't one of the original numbers (2, 4) — what does $\color{darkorange}{3!}$ mean?
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write 3! explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Did I surmise correctly?
## Red underline
2. Unquestionably, $\color{red}{\dfrac{8 \times 7 \times 6}{3!} = 8 × 7}$. But how can I construe and intuit $\color{red}{8 × 7}$ DIRECTLY? Note that $\color{red}{7 } $ wasn't one of the original numbers of 3 or 8. What does $\color{red}{7 } $ mean?
**_Here's my surmisal._** You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (David Patrick, p 38 bottom), the answer ought be 8 × 7 × 6??? Why isn't there 6?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
>
>![Image alt text](https://math.codidact.com/uploads/t7nrZLrLSsEu2HAspWbrpx4E)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.
## Examples 3 and 4
3. I know [the ways to form a committee of 2 from 8](https://mathhelpforum.com/threads/calculate-ways-to-form-a-committee-of-3-from-8-directly-without-%C3%B7.295777/post-975751) $= \dbinom8 2 = \dfrac{8\cdot7}{2!} = \color{violet}{4 × 7}.$ But how can I construe and intuit $\color{violet}{4 × 7}$ DIRECTLY? Note that $\color{violet}{4, 7}$ aren't the original numbers of 2, 8.
4. I know [the ways to form a committee of 3 from 10](https://mathforums.com/threads/calculate-ways-to-form-a-committee-of-3-from-8-directly-without-%C3%B7.362347/post-664313) $= \dbinom {10} 3 = \dfrac{10\cdot9\cdot8}{3!} = \color{springgreen}{10 × 3 × 4}.$ But how can I construe and intuit $\color{springgreen}{10 × 3 × 4}$ DIRECTLY? I'm surmising that $\color{springgreen}{10}$ refers to the original 10, and $\color{springgreen}{3}$ to the chosen 3. But what does $\color{springgreen}{4}$ mean?

I grok, am NOT asking about, the answers below. **Rather — how can I deduce and intuit the multiplicands and multiplicators $\le 10$, resulting from simplifying $\dbinom m n$ DIRECTLY? WITHOUT division or factorials!**
## Orange underline
1. Unquestionably, $\color{darkorange}{4 \times 3/2 = 3!}$ But how can I construe and intuit $\color{darkorange}{3!}$ DIRECTLY? Note that $\color{darkorange}{3!}$ wasn't one of the original numbers (2, 4) — what does $\color{darkorange}{3!}$ mean?
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write 3! explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Did I surmise correctly?
## Red underline
2. Unquestionably, $\color{red}{\dfrac{8 \times 7 \times 6}{3!} = 8 × 7}$. But how can I construe and intuit $\color{red}{8 × 7}$ DIRECTLY? Note that $\color{red}{7 } $ wasn't one of the original numbers of 3 or 8. What does $\color{red}{7 } $ mean?
**_Here's my surmisal._** You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (David Patrick, p 38 bottom), the answer ought be 8 × 7 × 6??? Why isn't there 6?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
>
>![Image alt text](https://math.codidact.com/uploads/t7nrZLrLSsEu2HAspWbrpx4E)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.
## Examples 3 and 4
3. I know [the ways to form a committee of 2 from 8](https://mathhelpforum.com/threads/calculate-ways-to-form-a-committee-of-3-from-8-directly-without-%C3%B7.295777/post-975751) $= \dbinom8 2 = \dfrac{8\cdot7}{2!} = \color{violet}{4 × 7}.$ But how can I construe and intuit $\color{violet}{4 × 7}$ DIRECTLY? Note that $\color{violet}{4, 7}$ aren't the original numbers of 2, 8.
4. I know [the ways to form a committee of 3 from 10](https://mathforums.com/threads/calculate-ways-to-form-a-committee-of-3-from-8-directly-without-%C3%B7.362347/post-664313) $= \dbinom {10} 3 = \dfrac{10\cdot9\cdot8}{3!} = \color{springgreen}{10 × 3 × 4}.$ But how can I construe and intuit $\color{springgreen}{10 × 3 × 4}$ DIRECTLY? I'm surmising that $\color{springgreen}{10}$ refers to the original 10, and $\color{springgreen}{3}$ to the chosen 3. But what does $\color{springgreen}{4}$ mean?

#8: Post edited by $user avatar$ DNB‭ · 2022-02-01T09:24:45Z (about 2 years ago)

Copy Link

Raw

Markdown

~~How to intuit, construe ways to form a m-member committee from p people directly WITHOUT division or factorials?~~

How to intuit, construe ways to form a m-member committee from p people directly, WITHOUT division or factorials?

I grok, am NOT asking about, the answers below. **Rather, how can I deduce and intuit the final answer DIRECTLY, WITHOUT division or factorials?**
## Orange underline
1. Unquestionably, $\color{darkorange}{4 \times 3/2 = 3!}$ But how can I construe and intuit $\color{darkorange}{3!}$ DIRECTLY? Note that $\color{darkorange}{3!}$ wasn't one of the original numbers (2, 4) — what does $\color{darkorange}{3!}$ mean?
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write 3! explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Did I surmise correctly?
## Red underline
2. Unquestionably, $\color{red}{\dfrac{8 \times 7 \times 6}{3!} = 8 × 7}$. But how can I construe and intuit $\color{red}{8 × 7}$ DIRECTLY? Note that $\color{red}{7 } $ wasn't one of the original numbers of 3 or 8. What does $\color{red}{7 } $ mean?
**_Here's my surmisal._** You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (David Patrick, p 38 bottom), the answer ought be 8 × 7 × 6??? Why isn't there 6?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
>
>![Image alt text](https://math.codidact.com/uploads/t7nrZLrLSsEu2HAspWbrpx4E)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.
## Examples 3 and 4
3. I know [the ways to form a committee of 2 from 8](https://mathhelpforum.com/threads/calculate-ways-to-form-a-committee-of-3-from-8-directly-without-%C3%B7.295777/post-975751) $= \dbinom8 2 = \dfrac{8\cdot7}{2!} = \color{violet}{4 × 7}.$ But again, how can I construe and intuit $\color{violet}{4 × 7}$ DIRECTLY? Note that $\color{violet}{4, 7}$ aren't the original numbers of 2, 8.
[4. I know [the ways to form a committee of 3 from 10](https://mathforums.com/threads/calculate-ways-to-form-a-committee-of-3-from-8-directly-without-%C3%B7.362347/post-664313) $= \dbinom {10} 3 = \dfrac{10\cdot9\cdot8}{3!} = \color{springgreen}{10 × 3 × 4}.$ But again, how can I construe and intuit $\color{springgreen}{10 × 3 × 4}$ DIRECTLY? I'm surmising that $\color{springgreen}{10}$ refers to the original 10, and $\color{springgreen}{3}$ to the chosen 3. But what does $\color{springgreen}{4}$ mean?

I grok, am NOT asking about, the answers below. **Rather, how can I deduce and intuit the final answer DIRECTLY, WITHOUT division or factorials?**
## Orange underline
1. Unquestionably, $\color{darkorange}{4 \times 3/2 = 3!}$ But how can I construe and intuit $\color{darkorange}{3!}$ DIRECTLY? Note that $\color{darkorange}{3!}$ wasn't one of the original numbers (2, 4) — what does $\color{darkorange}{3!}$ mean?
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write 3! explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Did I surmise correctly?
## Red underline
2. Unquestionably, $\color{red}{\dfrac{8 \times 7 \times 6}{3!} = 8 × 7}$. But how can I construe and intuit $\color{red}{8 × 7}$ DIRECTLY? Note that $\color{red}{7 } $ wasn't one of the original numbers of 3 or 8. What does $\color{red}{7 } $ mean?
**_Here's my surmisal._** You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (David Patrick, p 38 bottom), the answer ought be 8 × 7 × 6??? Why isn't there 6?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
>
>![Image alt text](https://math.codidact.com/uploads/t7nrZLrLSsEu2HAspWbrpx4E)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.
## Examples 3 and 4
3. I know [the ways to form a committee of 2 from 8](https://mathhelpforum.com/threads/calculate-ways-to-form-a-committee-of-3-from-8-directly-without-%C3%B7.295777/post-975751) $= \dbinom8 2 = \dfrac{8\cdot7}{2!} = \color{violet}{4 × 7}.$ But how can I construe and intuit $\color{violet}{4 × 7}$ DIRECTLY? Note that $\color{violet}{4, 7}$ aren't the original numbers of 2, 8.
4. I know [the ways to form a committee of 3 from 10](https://mathforums.com/threads/calculate-ways-to-form-a-committee-of-3-from-8-directly-without-%C3%B7.362347/post-664313) $= \dbinom {10} 3 = \dfrac{10\cdot9\cdot8}{3!} = \color{springgreen}{10 × 3 × 4}.$ But how can I construe and intuit $\color{springgreen}{10 × 3 × 4}$ DIRECTLY? I'm surmising that $\color{springgreen}{10}$ refers to the original 10, and $\color{springgreen}{3}$ to the chosen 3. But what does $\color{springgreen}{4}$ mean?

#7: Post edited by $user avatar$ DNB‭ · 2022-02-01T09:22:28Z (about 2 years ago)

Copy Link

Raw

Markdown

Why ways to pick a 2-person committee from 4 people $3!$? Why aren't ways to form a 3-member committee from 8 people $8 \times 7 \times 6$?

How to intuit, construe ways to form a m-member committee from p people directly WITHOUT division or factorials?

I grok, am NOT asking about, the answers below. **Rather, how can I calculate the final answer DIRECTLY, without division?**
## Orange underline
~~1. Unquestionably, $\color{darkorange}{4 \times 3/2} = 3!$ But how can I construe 3! DIRECTLY _WITHOUT DIVISION_? What does 3! mean?~~
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write 3! explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Is this correct?
## Red underline
~~2. Unquestionably, ${\color{red}{\dfrac{8 \times 7 \times 6}{3!}}}$ = 8 × 7. But how can I construe 8 × 7 DIRECTLY _WITHOUT DIVISION_? What does 8 × 7 mean?~~
**_Here's my surmisal._** You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (p 38 bottom), the answer ought be 8 × 7 × 6??? Why isn't there 6?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
![Image alt text](https://math.codidact.com/uploads/t7nrZLrLSsEu2HAspWbrpx4E)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.

I grok, am NOT asking about, the answers below. **Rather, how can I deduce and intuit the final answer DIRECTLY, WITHOUT division or factorials?**
## Orange underline
1. Unquestionably, $\color{darkorange}{4 \times 3/2 = 3!}$ But how can I construe and intuit $\color{darkorange}{3!}$ DIRECTLY? Note that $\color{darkorange}{3!}$ wasn't one of the original numbers (2, 4) — what does $\color{darkorange}{3!}$ mean?
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write 3! explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Did I surmise correctly?
## Red underline
2. Unquestionably, $\color{red}{\dfrac{8 \times 7 \times 6}{3!} = 8 × 7}$. But how can I construe and intuit $\color{red}{8 × 7}$ DIRECTLY? Note that $\color{red}{7 } $ wasn't one of the original numbers of 3 or 8. What does $\color{red}{7 } $ mean?
**_Here's my surmisal._** You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (David Patrick, p 38 bottom), the answer ought be 8 × 7 × 6??? Why isn't there 6?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
>
>![Image alt text](https://math.codidact.com/uploads/t7nrZLrLSsEu2HAspWbrpx4E)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.
## Examples 3 and 4
3. I know [the ways to form a committee of 2 from 8](https://mathhelpforum.com/threads/calculate-ways-to-form-a-committee-of-3-from-8-directly-without-%C3%B7.295777/post-975751) $= \dbinom8 2 = \dfrac{8\cdot7}{2!} = \color{violet}{4 × 7}.$ But again, how can I construe and intuit $\color{violet}{4 × 7}$ DIRECTLY? Note that $\color{violet}{4, 7}$ aren't the original numbers of 2, 8.
[4. I know [the ways to form a committee of 3 from 10](https://mathforums.com/threads/calculate-ways-to-form-a-committee-of-3-from-8-directly-without-%C3%B7.362347/post-664313) $= \dbinom {10} 3 = \dfrac{10\cdot9\cdot8}{3!} = \color{springgreen}{10 × 3 × 4}.$ But again, how can I construe and intuit $\color{springgreen}{10 × 3 × 4}$ DIRECTLY? I'm surmising that $\color{springgreen}{10}$ refers to the original 10, and $\color{springgreen}{3}$ to the chosen 3. But what does $\color{springgreen}{4}$ mean?

#6: Post edited by $user avatar$ DNB‭ · 2022-01-26T04:56:09Z (about 2 years ago)

Copy Link

Raw

Markdown

I grok, am NOT asking about, the answers below. **Rather, how can I calculate the final answer DIRECTLY, without division?**
## Orange underline
~~Unquestionably, $\color{darkorange}{4 \times 3/2} = 3!$ But how can I construe 3! DIRECTLY _WITHOUT DIVISION_? What does 3! mean?~~
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write $3!$ explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Is this correct?
## Red underline
~~Unquestionably, ${\color{red}{\dfrac{8 \times 7 \times 6}{3!}}} = 8 \times 7$. But how can I construe 8 × 7 DIRECTLY _WITHOUT DIVISION_? What does 8 × 7 mean?~~
**_Here's my surmisal._** You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (p 38 bottom), the answer ought be 8 × 7 × 6??? Why isn't there 6?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
![Image alt text](https://math.codidact.com/uploads/t7nrZLrLSsEu2HAspWbrpx4E)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.

I grok, am NOT asking about, the answers below. **Rather, how can I calculate the final answer DIRECTLY, without division?**
## Orange underline
1. Unquestionably, $\color{darkorange}{4 \times 3/2} = 3!$ But how can I construe 3! DIRECTLY _WITHOUT DIVISION_? What does 3! mean?
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write 3! explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Is this correct?
## Red underline
2. Unquestionably, ${\color{red}{\dfrac{8 \times 7 \times 6}{3!}}}$ = 8 × 7. But how can I construe 8 × 7 DIRECTLY _WITHOUT DIVISION_? What does 8 × 7 mean?
**_Here's my surmisal._** You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (p 38 bottom), the answer ought be 8 × 7 × 6??? Why isn't there 6?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
![Image alt text](https://math.codidact.com/uploads/t7nrZLrLSsEu2HAspWbrpx4E)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.

#5: Post edited by $user avatar$ DNB‭ · 2022-01-26T04:46:12Z (about 2 years ago)

Copy Link

Raw

Markdown

I grok, am NOT asking about, the answers below. **Rather, how can I calculate the final answer DIRECTLY, without factorials?**
## Orange underline
~~Unquestionably, $\color{darkorange}{4 \times 3/2} = 3!$ But how can I construe 3! directly WITHOUT FACTORIALS? What does 3! mean?~~
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write $3!$ explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Is this correct?
## Red underline
~~Unquestionably, ${\color{red}{\dfrac{8 \times 7 \times 6}{3!}}} = 8 \times 7$. But how can I construe 8 × 7 directly, WITHOUT FACTORIALS? What does 8 × 7 mean?~~
**_Here's my surmisal._** You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (p 38 bottom), the answer ought be 8 × 7 × 6??? Why isn't there 6?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
![Image alt text](https://math.codidact.com/uploads/t7nrZLrLSsEu2HAspWbrpx4E)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.

I grok, am NOT asking about, the answers below. **Rather, how can I calculate the final answer DIRECTLY, without division?**
## Orange underline
Unquestionably, $\color{darkorange}{4 \times 3/2} = 3!$ But how can I construe 3! DIRECTLY _WITHOUT DIVISION_? What does 3! mean?
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write $3!$ explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Is this correct?
## Red underline
Unquestionably, ${\color{red}{\dfrac{8 \times 7 \times 6}{3!}}} = 8 \times 7$. But how can I construe 8 × 7 DIRECTLY _WITHOUT DIVISION_? What does 8 × 7 mean?
**_Here's my surmisal._** You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (p 38 bottom), the answer ought be 8 × 7 × 6??? Why isn't there 6?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
![Image alt text](https://math.codidact.com/uploads/t7nrZLrLSsEu2HAspWbrpx4E)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.

#4: Post edited by $user avatar$ DNB‭ · 2022-01-26T04:44:54Z (about 2 years ago)

Copy Link

Raw

Markdown

I grok, am NOT asking about, the answers below. Rather, how can I calculate the final answer directly without any multiplication?
~~## Red underline~~
~~Unquestionably, $\color{red}{4 \times 3/2} = 3!$ But why's $3!$ the answer? What does $3!$ mean?~~
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write $3!$ explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Is this correct?
~~## Purple underline~~
~~Unquestionably, ${\color{darkviolet}{\dfrac{8 \times 7 \times 6}{3!}}} = 8 \times 7$. But why's $8 \times 7$ the answer? What does $8 \times 7$ mean?~~
**_Here's my surmisal._** You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (p 38 bottom), the answer ought be $8 \times 7 \times 6$??? Why isn't there 6?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
~~![Image alt text](https://math.codidact.com/uploads/ZiZjtsBPYFoQzu1EA1xq78Vz)~~
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.

I grok, am NOT asking about, the answers below. **Rather, how can I calculate the final answer DIRECTLY, without factorials?**
## Orange underline
Unquestionably, $\color{darkorange}{4 \times 3/2} = 3!$ But how can I construe 3! directly WITHOUT FACTORIALS? What does 3! mean?
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write $3!$ explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Is this correct?
## Red underline
Unquestionably, ${\color{red}{\dfrac{8 \times 7 \times 6}{3!}}} = 8 \times 7$. But how can I construe 8 × 7 directly, WITHOUT FACTORIALS? What does 8 × 7 mean?
**_Here's my surmisal._** You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (p 38 bottom), the answer ought be 8 × 7 × 6??? Why isn't there 6?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
![Image alt text](https://math.codidact.com/uploads/t7nrZLrLSsEu2HAspWbrpx4E)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.

#3: Question closed by $user avatar$ Peter Taylor‭ · 2022-01-25T09:20:24Z (about 2 years ago)

Copy Link

Raw

Markdown

#2: Post edited by $user avatar$ DNB‭ · 2022-01-25T05:47:57Z (about 2 years ago)

Copy Link

Raw

Markdown

I grok, am NOT asking about, the answers below. Rather, how can I calculate the final answer directly without any multiplication?
## Red underline
~~Unquestionably, $\color{red}{4 \times 3/2} = 3!$ But why's 3! the answer? What does 3! represent here?~~
Here's my surmisal. [Blitzstein's solution hints to this calculation, but he didn't write $3!$ explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then there are 3 people left, so there are 3 ways to choose the second person in your 2-person committee? Is this correct?
## Purple underline
~~Unquestionably, ${\color{darkviolet}{\dfrac{8 \times 7 \times 6}{3!}}} = 8 \times 7$. But why's $8 \times 7$ the answer? What does $8 \times 7$ represent here?~~
Here's my surmisal. There are 8 ways to pick the 1st committee member, and 7 ways to pick the 2nd member. But then wouldn't there be 6 ways to pick the 3rd member? By this Constructive Counting (p 38 bottom), the answer ought be $8 \times 7 \times 6$???
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
![Image alt text](https://math.codidact.com/uploads/ZiZjtsBPYFoQzu1EA1xq78Vz)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.

I grok, am NOT asking about, the answers below. Rather, how can I calculate the final answer directly without any multiplication?
## Red underline
Unquestionably, $\color{red}{4 \times 3/2} = 3!$ But why's $3!$ the answer? What does $3!$ mean?
**_Here's my surmisal._** [Blitzstein's solution hints to this calculation, but he didn't write $3!$ explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Is this correct?
## Purple underline
Unquestionably, ${\color{darkviolet}{\dfrac{8 \times 7 \times 6}{3!}}} = 8 \times 7$. But why's $8 \times 7$ the answer? What does $8 \times 7$ mean?
**_Here's my surmisal._** You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (p 38 bottom), the answer ought be $8 \times 7 \times 6$??? Why isn't there 6?
>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the
order in which we choose the 2 people doesn't matter)?
![Image alt text](https://math.codidact.com/uploads/ZiZjtsBPYFoQzu1EA1xq78Vz)
David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon), PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.

#1: Initial revision by $user avatar$ DNB‭ · 2022-01-25T05:46:05Z (about 2 years ago)

Copy Link

Raw

Markdown

Why ways to pick a 2-person committee from 4 people $3!$? Why aren't ways to form a 3-member committee from 8 people $8 \times 7 \times 6$?

I grok, am NOT asking about, the answers below. Rather, how can I calculate the final answer directly without any multiplication?

## Red underline

Unquestionably, $\color{red}{4 \times 3/2} = 3!$ But why's 3! the answer? What does 3! represent here?

Here's my surmisal. [Blitzstein's solution hints to this calculation, but he didn't write $3!$ explicitly](https://math.codidact.com/posts/282608). You fix the first person. Then there are 3 people left, so there are 3 ways to choose the second person in your 2-person committee? Is this correct?

## Purple underline

Unquestionably, ${\color{darkviolet}{\dfrac{8 \times 7 \times 6}{3!}}} = 8 \times 7$. But why's $8 \times 7$ the answer? What does $8 \times 7$  represent here? 

Here's my surmisal. There are 8 ways to pick the 1st committee member, and 7 ways to pick the 2nd member. But then wouldn't there be 6 ways to pick the 3rd member? By this Constructive Counting (p 38 bottom), the answer ought be $8 \times 7 \times 6$??? 


>**Problem 4.1:**
>
>(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the 
order in which we choose the 2 people doesn't matter)? 


![Image alt text](https://math.codidact.com/uploads/ZiZjtsBPYFoQzu1EA1xq78Vz)

David Patrick, [BS Math & Computer Science, MS Math (Carnegie Mellon),  PhD Math (MIT)](https://artofproblemsolving.com/wiki/index.php/David_Patrick). *Introduction to Counting & Probability* (2005), pp 66-7.

combinatorics

Communities

Post History