Post History

40%

+0 −1

Q&A If C = Calvin wins the match, and $X \thicksim Bin(2, p) =$ how many of the first 2 games he wins — then why P(C|X = 1) = P(C)?

1 answer · posted 2y ago by DNB‭ · last activity 2y ago by Peter Taylor‭

Question probability

#2: Post edited by $user avatar$ DNB‭ · 2022-01-05T05:23:09Z (over 2 years ago)

Copy Link

Raw

Markdown

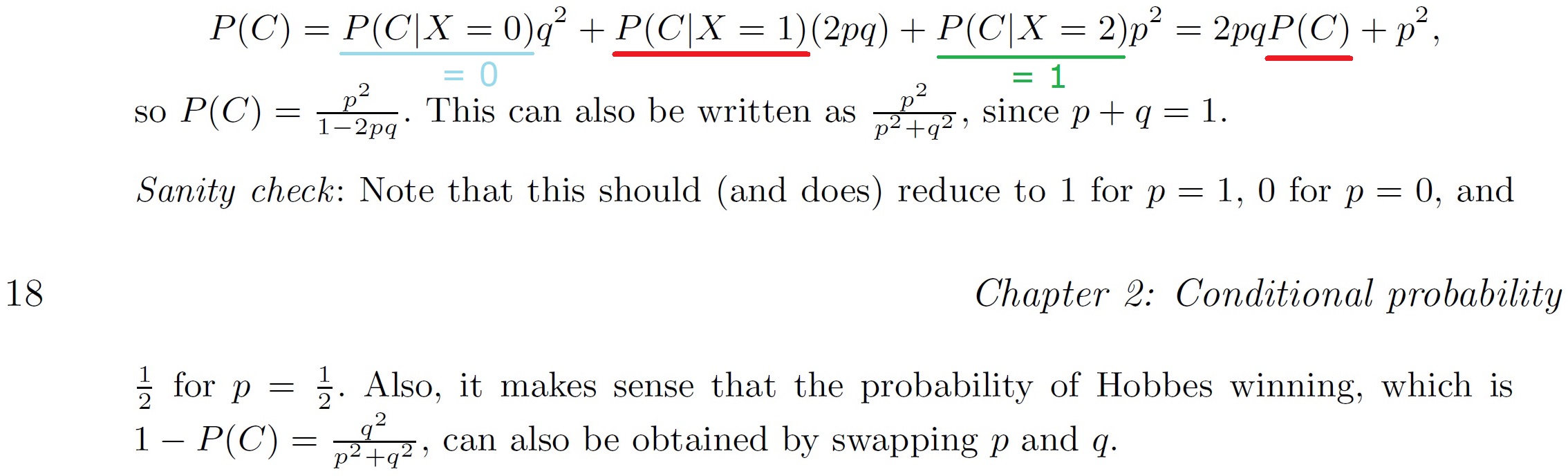
~~The author's solution doesn't expatiate why $\color{red}{P(C|X = 1) = P(C)}$?~~
>50. Calvin and Hobbes play a match consisting of a series of games, where Calvin has
probability p of winning each game (independently). They play with a "win by two" rule: the first player to win two games more than his opponent wins the match. Find
the probability that Calvin wins the match (in terms of p), in two different ways:
>
>(a) by conditioning, using the law of total probability.
>
>(b) by interpreting the problem as a gambler's ruin problem.
>
>## Solution:
>
>(a) Let C be the event that Calvin wins the match, $X \thicksim Bin(2, p)$ be how many of the first 2 games he wins, and $q = 1 - p$. Then
>
>![Image alt text](https://math.codidact.com/uploads/c2eDuHry2utfhsM2X9FpRFmk)
Blitzstein, *Introduction to Probability* (2019 2 edn), Chapter 2, Exercise 50, p 94.
p 17 in the publicly downloadable PDF of curbed solutions.

The author's solution doesn't expatiate why $\color{red}{P(C|X = 1) = P(C)}$? [This similar question on Math Stack Exchange](https://math.stackexchange.com/q/4288685) has 0 answers, as at 4 January 2022.
>50. Calvin and Hobbes play a match consisting of a series of games, where Calvin has
probability p of winning each game (independently). They play with a "win by two" rule: the first player to win two games more than his opponent wins the match. Find
the probability that Calvin wins the match (in terms of p), in two different ways:
>
>(a) by conditioning, using the law of total probability.
>
>(b) by interpreting the problem as a gambler's ruin problem.
>
>## Solution:
>
>(a) Let C be the event that Calvin wins the match, $X \thicksim Bin(2, p)$ be how many of the first 2 games he wins, and $q = 1 - p$. Then
>
>![Image alt text](https://math.codidact.com/uploads/c2eDuHry2utfhsM2X9FpRFmk)
Blitzstein, *Introduction to Probability* (2019 2 edn), Chapter 2, Exercise 50, p 94.
p 17 in the publicly downloadable PDF of curbed solutions.

#1: Initial revision by $user avatar$ DNB‭ · 2021-12-31T08:24:45Z (over 2 years ago)

Copy Link

Raw

Markdown

If C = Calvin wins the match, and $X \thicksim Bin(2, p) =$ how many of the first 2 games he wins   — then why P(C|X = 1) = P(C)?

The author's solution doesn't expatiate why $\color{red}{P(C|X = 1) = P(C)}$? 

>50. Calvin and Hobbes play a match consisting of a series of games, where Calvin has
probability p of winning each game (independently). They play with a "win by two" rule: the first player to win two games more than his opponent wins the match. Find
the probability that Calvin wins the match (in terms of p), in two different ways:
>
>(a) by conditioning, using the law of total probability.
>
>(b) by interpreting the problem as a gambler's ruin problem.
>
>## Solution:
>
>(a) Let C be the event that Calvin wins the match, $X \thicksim Bin(2, p)$ be how many of the first 2 games he wins, and $q = 1 - p$. Then
>
>![Image alt text](https://math.codidact.com/uploads/c2eDuHry2utfhsM2X9FpRFmk)

Blitzstein, *Introduction to Probability* (2019 2 edn), Chapter 2, Exercise 50, p 94.    
p 17 in the publicly downloadable PDF of curbed solutions.

probability

Communities

Post History