Post History
#3: Post edited
by
DNB
·
2021-12-24T06:46:37Z (over 2 years ago)
$\color{red}{P(B|M)} = 1 - \color{forestgreen}{P(A|M)}$?
- In general, does $\color{forestgreen}{P(A|M)} + \color{red}{P(B|M)} = 1$?
In this question, $\color{forestgreen}{P(A|M)} + \color{red}{P(B|M)} = 1$. The author's solution doesn't unfurl how he computed $\color{red}{P(B|M)}$. Can I simply subtract as $\color{red}{P(B|M)} = 1 - \color{forestgreen}{P(A|M)}$? I am hankering to avoid calculating $\color{red}{P(B|M)}$ by applying Bayes's Rule a second time.- >25. A crime is committed by one of two suspects, A and B. Initially, there is equal
- evidence against both of them. In further investigation at the crime scene, it is found
- that the guilty party had a blood type found in 10% of the population. Suspect A does
- match this blood type, whereas the blood type of Suspect B is unknown.
- >
- >(a) Given this new information, what is the probability that A is the guilty party?
- >
- >(b) Given this new information, what is the probability that B's blood type matches
- that found at the crime scene?
- >
- >## Solution:
- >
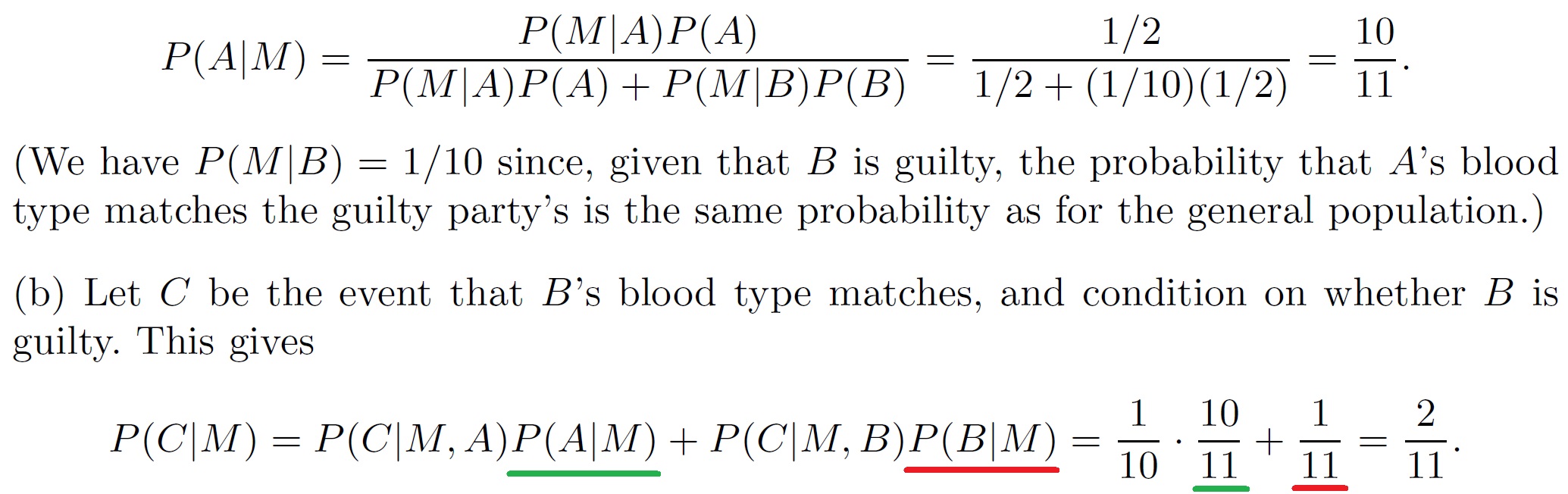
- >(a) Let M be the event that A's blood type matches the guilty party's and for brevity,
- write A for "A is guilty" and B for "B is guilty". By Bayes' Rule,
- >
- >
- Blitzstein, *Introduction to Probability* (2019 2 edn), Ch 2, Exercise 25, p 87. p 12 in the publicly downloadable PDF of curbed solutions.
- In this question, $\color{forestgreen}{P(A|M)} + \color{red}{P(B|M)} = 1$. But the author's solution didn't annunciate this, and doesn't unfurl how to compute $\color{red}{P(B|M)}$. Can I simply subtract as $\color{red}{P(B|M)} = 1 - \color{forestgreen}{P(A|M)}$? I hanker to avoid calculating $\color{red}{P(B|M)}$ by applying Bayes's Rule a second time.
- >25. A crime is committed by one of two suspects, A and B. Initially, there is equal
- evidence against both of them. In further investigation at the crime scene, it is found
- that the guilty party had a blood type found in 10% of the population. Suspect A does
- match this blood type, whereas the blood type of Suspect B is unknown.
- >
- >(a) Given this new information, what is the probability that A is the guilty party?
- >
- >(b) Given this new information, what is the probability that B's blood type matches
- that found at the crime scene?
- >
- >## Solution:
- >
- >(a) Let M be the event that A's blood type matches the guilty party's and for brevity,
- write A for "A is guilty" and B for "B is guilty". By Bayes' Rule,
- >
- >
- Blitzstein, *Introduction to Probability* (2019 2 edn), Ch 2, Exercise 25, p 87. p 12 in the publicly downloadable PDF of curbed solutions.
#2: Post edited
by
DNB
·
2021-12-23T09:11:46Z (over 2 years ago)
- In this question, $\color{forestgreen}{P(A|M)} + \color{red}{P(B|M)} = 1$. The author's solution doesn't unfurl how he computed $\color{red}{P(B|M)}$. Can I simply subtract as $\color{red}{P(B|M)} = 1 - \color{forestgreen}{P(A|M)}$? I am hankering to avoid calculating $\color{red}{P(B|M)}$ by applying Bayes's Rule a second time.
- >25. A crime is committed by one of two suspects, A and B. Initially, there is equal
- evidence against both of them. In further investigation at the crime scene, it is found
- that the guilty party had a blood type found in 10% of the population. Suspect A does
- match this blood type, whereas the blood type of Suspect B is unknown.
- >
- >(a) Given this new information, what is the probability that A is the guilty party?
- >
- >(b) Given this new information, what is the probability that B's blood type matches
- that found at the crime scene?
- >
- >## Solution:
- >
- >(a) Let M be the event that A's blood type matches the guilty party's and for brevity,
- write A for "A is guilty" and B for "B is guilty". By Bayes' Rule,
- >
- >
Blitzstein, *Introduction to Probability* (2019 2 edn), Ch 2, Exercise 25, p 87. p 12 in Student's Solution Manual.
- In this question, $\color{forestgreen}{P(A|M)} + \color{red}{P(B|M)} = 1$. The author's solution doesn't unfurl how he computed $\color{red}{P(B|M)}$. Can I simply subtract as $\color{red}{P(B|M)} = 1 - \color{forestgreen}{P(A|M)}$? I am hankering to avoid calculating $\color{red}{P(B|M)}$ by applying Bayes's Rule a second time.
- >25. A crime is committed by one of two suspects, A and B. Initially, there is equal
- evidence against both of them. In further investigation at the crime scene, it is found
- that the guilty party had a blood type found in 10% of the population. Suspect A does
- match this blood type, whereas the blood type of Suspect B is unknown.
- >
- >(a) Given this new information, what is the probability that A is the guilty party?
- >
- >(b) Given this new information, what is the probability that B's blood type matches
- that found at the crime scene?
- >
- >## Solution:
- >
- >(a) Let M be the event that A's blood type matches the guilty party's and for brevity,
- write A for "A is guilty" and B for "B is guilty". By Bayes' Rule,
- >
- >
- Blitzstein, *Introduction to Probability* (2019 2 edn), Ch 2, Exercise 25, p 87. p 12 in the publicly downloadable PDF of curbed solutions.
#1: Initial revision
by
DNB
·
2021-12-23T09:08:25Z (over 2 years ago)
$\color{red}{P(B|M)} = 1 - \color{forestgreen}{P(A|M)}$?
In this question, $\color{forestgreen}{P(A|M)} + \color{red}{P(B|M)} = 1$. The author's solution doesn't unfurl how he computed $\color{red}{P(B|M)}$. Can I simply subtract as $\color{red}{P(B|M)} = 1 - \color{forestgreen}{P(A|M)}$? I am hankering to avoid calculating $\color{red}{P(B|M)}$ by applying Bayes's Rule a second time.
>25. A crime is committed by one of two suspects, A and B. Initially, there is equal
evidence against both of them. In further investigation at the crime scene, it is found
that the guilty party had a blood type found in 10% of the population. Suspect A does
match this blood type, whereas the blood type of Suspect B is unknown.
>
>(a) Given this new information, what is the probability that A is the guilty party?
>
>(b) Given this new information, what is the probability that B's blood type matches
that found at the crime scene?
>
>## Solution:
>
>(a) Let M be the event that A's blood type matches the guilty party's and for brevity,
write A for "A is guilty" and B for "B is guilty". By Bayes' Rule,
>
>
Blitzstein, *Introduction to Probability* (2019 2 edn), Ch 2, Exercise 25, p 87. p 12 in Student's Solution Manual.