Post History
#4: Post edited
by
DNB
·
2021-08-11T08:36:43Z (over 3 years ago)
How to vaticinate calculating $w_{k + 1} = w_1(1 + \dfrac{1- p}{p} + \dots + (\dfrac{1- p}{p})^k)$ separately for $p = 1 - p$ and $peq 1 - p$?
- How to vaticinate calculating $w_{k + 1} = w_1(1 + \dfrac{1- p}{p} + \dots + [\dfrac{1- p}{p}]^k)$ separately for $p = 1 - p$ and $p
- eq 1 - p$?
Please see the orange, green, red underlines. 1. Why does $\color{limegreen}{w_{k + 1} = w_1(1 + \dfrac{1- p}{p} + \dots + (\dfrac{1- p}{p})^k)}$ beget the two cases of $\color{red}p = 1 - p$ and $\color{red}peq 1 - p$?2. Even after reading the solution, I wouldn't have prognosticated to calculate separately $\color{limegreen}{w_{k + 1} = w_1(1 + \dfrac{1- p}{p} + \dots + (\dfrac{1- p}{p})^k)}$ for the two cases $\color{red}p = 1 - p$ and $\color{red}peq 1 - p$. How would you divine this?-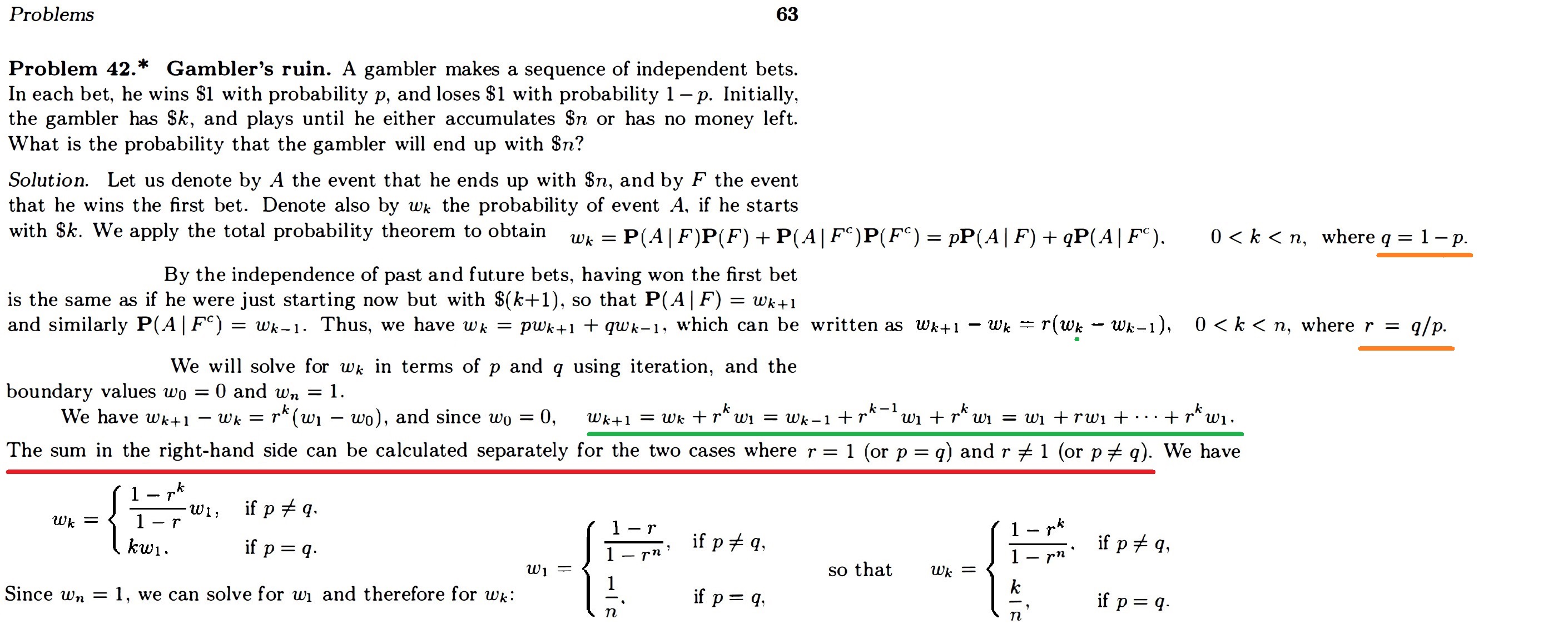 
- Tsitsiklis, *Introduction to Probability* (2008 2e), p 63.
- Please see the orange, green, red underlines. 1. Why does $\color{limegreen}{w_{k + 1} = w_1(1 + \dfrac{1- p}{p} + \dots + p\dfrac{1- p}{p}]^k)}$ beget the two cases of $\color{red}p = 1 - p$ and $\color{red}p
- eq 1 - p$?
- 2. Even after reading the solution, I wouldn't have prognosticated to calculate separately $\color{limegreen}{w_{k + 1} = w_1(1 + \dfrac{1- p}{p} + \dots + p\dfrac{1- p}{p})^k]}$ for the two cases $\color{red}p = 1 - p$ and $\color{red}p
- eq 1 - p$. How would you divine this?
- 
- Tsitsiklis, *Introduction to Probability* (2008 2e), p 63.
#3: Post edited
by
DNB
·
2021-08-11T08:36:02Z (over 3 years ago)
How to vaticinate calculating $w_{k + 1} = w_1(1 + \dfrac{1- p}{p} + \dots + (\dfrac{1- p}{p})^k$ separately for $p = 1 - p$ and $peq 1 - p$?
- How to vaticinate calculating $w_{k + 1} = w_1(1 + \dfrac{1- p}{p} + \dots + (\dfrac{1- p}{p})^k)$ separately for $p = 1 - p$ and $p
- eq 1 - p$?
#2: Post edited
by
DNB
·
2021-08-11T08:35:46Z (over 3 years ago)
How would you vaticinate to calculate $w_{k + 1} = w_1(1 + \dfrac{1- p}{p} + \dots + (\dfrac{1- p}{p} separately for $p = 1 - p$ and $peq 1 - p$?
- How to vaticinate calculating $w_{k + 1} = w_1(1 + \dfrac{1- p}{p} + \dots + (\dfrac{1- p}{p})^k$ separately for $p = 1 - p$ and $p
- eq 1 - p$?
#1: Initial revision
by
DNB
·
2021-08-11T08:35:07Z (over 3 years ago)
How would you vaticinate to calculate $w_{k + 1} = w_1(1 + \dfrac{1- p}{p} + \dots + (\dfrac{1- p}{p} separately for $p = 1 - p$ and $p \neq 1 - p$?
Please see the orange, green, red underlines. 1. Why does $\color{limegreen}{w_{k + 1} = w_1(1 + \dfrac{1- p}{p} + \dots + (\dfrac{1- p}{p})^k)}$ beget the two cases of $\color{red}p = 1 - p$ and $\color{red}p \neq 1 - p$?
2. Even after reading the solution, I wouldn't have prognosticated to calculate separately $\color{limegreen}{w_{k + 1} = w_1(1 + \dfrac{1- p}{p} + \dots + (\dfrac{1- p}{p})^k)}$ for the two cases $\color{red}p = 1 - p$ and $\color{red}p \neq 1 - p$. How would you divine this?
Tsitsiklis, *Introduction to Probability* (2008 2e), p 63.