Post History
#2: Post edited
by
DNB
·
2021-08-11T08:26:15Z (over 3 years ago)
- Please see the $r^k$ underlined in red, which is $(\frac{1 - p}{p})^k$ as defined by the green underlines.
- 1. How do you deduce that the exponent must be $k$? Why isn't the exponent $k + 1$?
2. Is this question related to [the Fence Post Error](https://betterexplained.com/articles/learning-how-to-count-avoiding-the-fencepost-problem/)? Have I committed it?-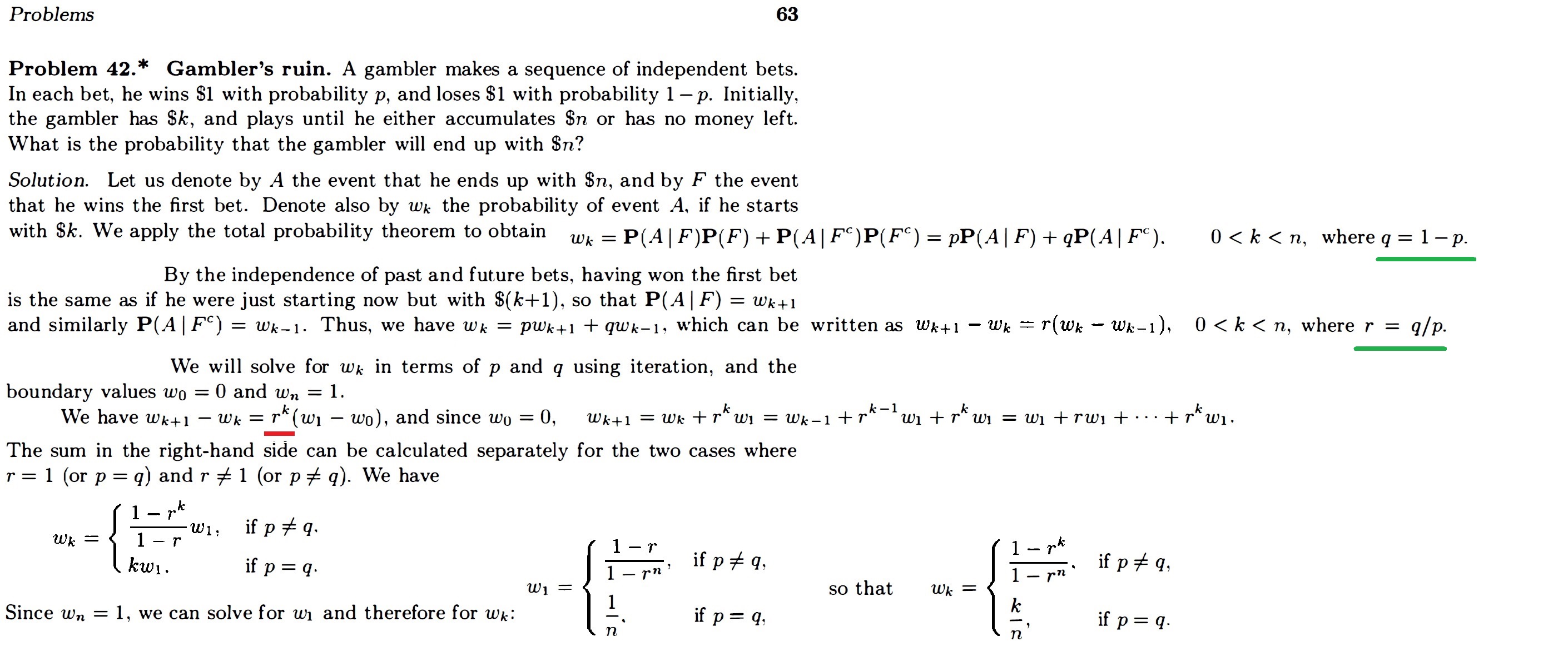 
- Tsitsiklis, *Introduction to Probability* (2008 2e), p 63.
- Please see the $r^k$ underlined in red, which is $(\frac{1 - p}{p})^k$ as defined by the green underlines.
- 1. How do you deduce that the exponent must be $k$? Why isn't the exponent $k + 1$?
- 2. Is this question related to [the Fence Post Error](https://mathenchant.wordpress.com/2017/11/16/impaled-on-a-fencepost/)? Have I committed it?
- 
- Tsitsiklis, *Introduction to Probability* (2008 2e), p 63.
#1: Initial revision
by
DNB
·
2021-08-11T08:23:25Z (over 3 years ago)
In $w_{k + 1} - w_k = (\frac{1 - p}{p})^{exponent}(w_1 - w_0)$, why isn't exponent $k + 1$?
Please see the $r^k$ underlined in red, which is $(\frac{1 - p}{p})^k$ as defined by the green underlines.
1. How do you deduce that the exponent must be $k$? Why isn't the exponent $k + 1$?
2. Is this question related to [the Fence Post Error](https://betterexplained.com/articles/learning-how-to-count-avoiding-the-fencepost-problem/)? Have I committed it?
Tsitsiklis, *Introduction to Probability* (2008 2e), p 63.