Post History
#6: Post edited
- The second quotation below uses the verb "shift" to describe how Monty Hall's opening the 98 unchosen doors (revealing a goat each) ""shifts" [boldening mine] that 99/100 chance to door #100"? The first quotation below uses "transfer". But how can probabilities "shift" or "transfer"?
- This analogy sounds featherbrained, but I hope it explains my bafflement. Imagine a doorman beside each door. Each time after the host opens an unchosen door (revealing a goat), each doorman walks over and congregates at another random unchosen door. After the host opens $n - 2$ unpicked doors, then $n - 2$ doormen will have congregated at the lone unchosen door. But probabilities aren't like doormen! From the $n - 2$ unchosen doors, they can't then "relocate" or "shift" to the lone unchosen door!
1. Judea Pearl, _The Book of Why _(2018). Page 181 of 402.- >
- > As you can see from Figure 6.1, Door Opened is a collider. Once we
- obtain information on this variable, all our probabilities become conditional
- on this information. But when we condition on a collider, we create a spurious
- dependence between its parents. The dependence is borne out in the
- probabilities: if you chose Door 1, the car location is twice as likely to be
- behind Door 2 as Door 1; if you chose Door 2, the car location is twice as
- likely to be behind Door 1.
- > It is a bizarre dependence for sure, one of a type that most of us are
- unaccustomed to. It is a dependence that has no cause. It does not involve
- physical communication between the producers and us. It does not involve
- mental telepathy. It is purely an artifact of Bayesian conditioning: a magical
- transfer of information without causality. Our minds rebel at this possibility
- because from earliest infancy, we have learned to associate correlation with
- causation. If a car behind us takes all the same turns that we do, we first think
- it is following us (causation!). We next think that we are going to the same
- place (i.e., there is a common cause behind each of our turns). But causeless
- correlation violates our common sense. Thus, the Monty Hall paradox is just
- like an optical illusion or a magic trick: it uses our own cognitive machinery
- to deceive us.
- > Why do I say that Monty Hall’s opening of Door 3 was a “transfer of
- information”? It didn’t, after all, provide any evidence about whether your
- initial choice of Door 1 was correct. You knew in advance that he was going
- to open a door that hid a goat, and so he did. No one should ask you to change
- your beliefs if you witness the inevitable. So how come your belief in Door 2
- has gone up from one-third to two-thirds?
- 2. [The Time Everyone “Corrected” the World’s Smartest Woman](https://priceonomics.com/the-time-everyone-corrected-the-worlds-smartest/)
- > Here’s another way to visualize this. Imagine that instead of three doors, Monty Hall presents you with 100 doors; behind 99 of them are goats, and behind one of them is the car. You select door #1, and your initial odds of winning the car are now 1/100:
- >
- > 
- >
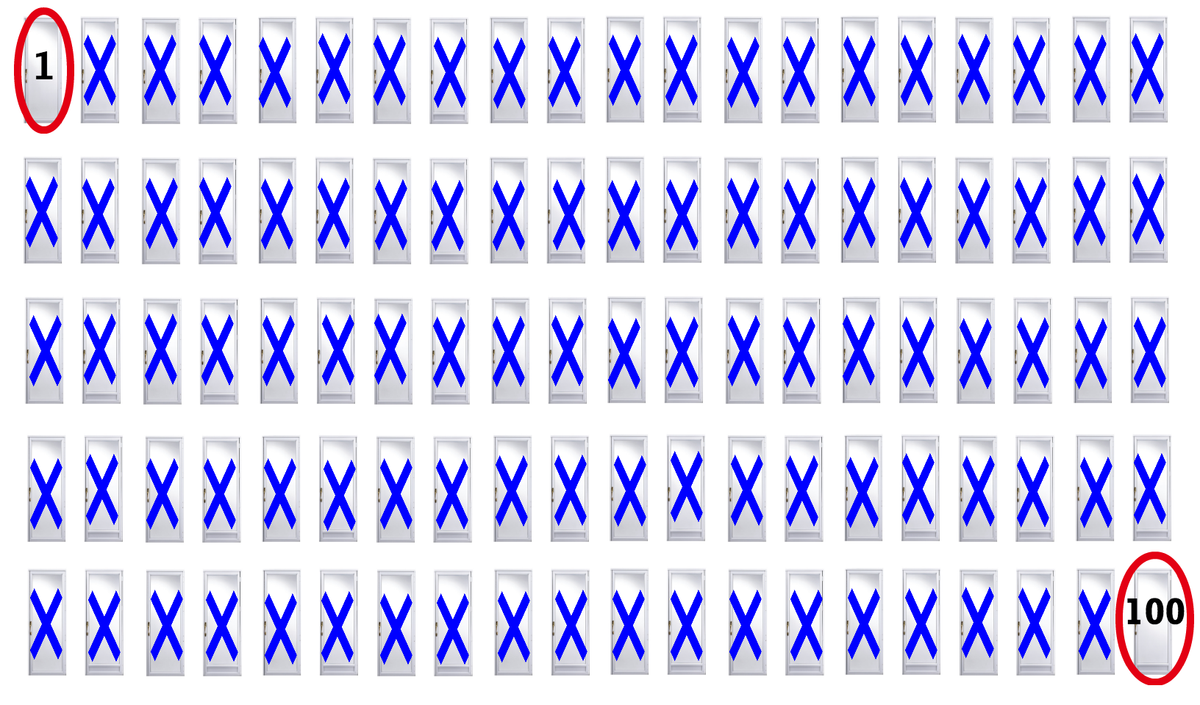
- > Then, let’s suppose that Monty Hall opens 98 of the other doors, revealing a goat behind each one. Now you’re left with two choices: keep door #1, or switch to door #100:
- >
- > 
- >
- > When you select door #1, there is a 99/100 chance that the car is behind one of the other doors. The fact that Monty Hall reveals 98 goats does not change these initial odds -- it merely **"shifts" [boldening mine]** that 99/100 chance to door #100. You can either stick with your original 1/100 odds pick, or switch to door #100, with a much higher probability of winning the car.
- 3. [by Robert Lea | Medium](https://medium.com/@roblea_63049/exploring-one-of-mathematics-most-enigmatic-puzzles-the-monty-hall-problem-f7b0beb8e1f1)
- 
- > At this point the switch shouldn’t seem attractive, there’s no benefit to changing. That all changes when Monty opens door C. This action means that there is then still a 2/3 chance that the car isn’t behind door A. This means there is a 2/3 chance the car is behind B or C. The opening of door C hasn’t affected the probabilities associated with door A but it has affected those associated with door B. It’s almost as if door B **must carry the full load [boldening mine]** of probabilities that was previously held by both B and C. This is because C isn’t chosen randomly. There is a 2/3 chance the car is behind the B&C pairing, that burden is left to B. Let’s look at that diagrammatically representing B & C as a pair with joint probabilities.
- 
- >Now let’s have Monty open door C again and see how that affects the probabilities.
- 
- >### The Hundred Doors Solution to The Monty Hall Problem.
- >
- >In this hypothetical version of the problem the contestant chooses not from 3 doors but from a hundred, for convenience we’ll label these doors 1–100 and say our contestant selects door 1. She has a 1/100 of selecting the door hiding the car and a 99/100 chance of not selecting a car. Let’s represent that with a stripped-down diagram. Again, black denotes the chance of selecting the car. Red the chance of not selecting the car.
- 
- >Now alternative universe Monty works his way through doors 2–100 careful revealing bearded goats until the bored contestant is left with two doors. Door 1 which she initially chose and door 2 the last remaining unchosen door. Here’s how the odds stack up at this point.
- 
- The second quotation below uses the verb "shift" to describe how Monty Hall's opening the 98 unchosen doors (revealing a goat each) ""shifts" [boldening mine] that 99/100 chance to door #100"? The first quotation below uses "transfer". But how can probabilities "shift" or "transfer"?
- This analogy sounds featherbrained, but I hope it explains my bafflement. Imagine a doorman beside each door. Each time after the host opens an unchosen door (revealing a goat), each doorman walks over and congregates at another random unchosen door. After the host opens $n - 2$ unpicked doors, then $n - 2$ doormen will have congregated at the lone unchosen door. But probabilities aren't like doormen! From the $n - 2$ unchosen doors, they can't then "relocate" or "shift" to the lone unchosen door!
- 1. Judea Pearl, _The Book of Why_ (2018). Page 181 of 402.
- >
- > As you can see from Figure 6.1, Door Opened is a collider. Once we
- obtain information on this variable, all our probabilities become conditional
- on this information. But when we condition on a collider, we create a spurious
- dependence between its parents. The dependence is borne out in the
- probabilities: if you chose Door 1, the car location is twice as likely to be
- behind Door 2 as Door 1; if you chose Door 2, the car location is twice as
- likely to be behind Door 1.
- > It is a bizarre dependence for sure, one of a type that most of us are
- unaccustomed to. It is a dependence that has no cause. It does not involve
- physical communication between the producers and us. It does not involve
- mental telepathy. It is purely an artifact of Bayesian conditioning: a magical
- transfer of information without causality. Our minds rebel at this possibility
- because from earliest infancy, we have learned to associate correlation with
- causation. If a car behind us takes all the same turns that we do, we first think
- it is following us (causation!). We next think that we are going to the same
- place (i.e., there is a common cause behind each of our turns). But causeless
- correlation violates our common sense. Thus, the Monty Hall paradox is just
- like an optical illusion or a magic trick: it uses our own cognitive machinery
- to deceive us.
- > Why do I say that Monty Hall’s opening of Door 3 was a “transfer of
- information”? It didn’t, after all, provide any evidence about whether your
- initial choice of Door 1 was correct. You knew in advance that he was going
- to open a door that hid a goat, and so he did. No one should ask you to change
- your beliefs if you witness the inevitable. So how come your belief in Door 2
- has gone up from one-third to two-thirds?
- 2. [The Time Everyone “Corrected” the World’s Smartest Woman](https://priceonomics.com/the-time-everyone-corrected-the-worlds-smartest/)
- > Here’s another way to visualize this. Imagine that instead of three doors, Monty Hall presents you with 100 doors; behind 99 of them are goats, and behind one of them is the car. You select door #1, and your initial odds of winning the car are now 1/100:
- >
- > 
- >
- > Then, let’s suppose that Monty Hall opens 98 of the other doors, revealing a goat behind each one. Now you’re left with two choices: keep door #1, or switch to door #100:
- >
- > 
- >
- > When you select door #1, there is a 99/100 chance that the car is behind one of the other doors. The fact that Monty Hall reveals 98 goats does not change these initial odds -- it merely **"shifts" [boldening mine]** that 99/100 chance to door #100. You can either stick with your original 1/100 odds pick, or switch to door #100, with a much higher probability of winning the car.
- 3. [by Robert Lea | Medium](https://medium.com/@roblea_63049/exploring-one-of-mathematics-most-enigmatic-puzzles-the-monty-hall-problem-f7b0beb8e1f1)
- 
- > At this point the switch shouldn’t seem attractive, there’s no benefit to changing. That all changes when Monty opens door C. This action means that there is then still a 2/3 chance that the car isn’t behind door A. This means there is a 2/3 chance the car is behind B or C. The opening of door C hasn’t affected the probabilities associated with door A but it has affected those associated with door B. It’s almost as if door B **must carry the full load [boldening mine]** of probabilities that was previously held by both B and C. This is because C isn’t chosen randomly. There is a 2/3 chance the car is behind the B&C pairing, that burden is left to B. Let’s look at that diagrammatically representing B & C as a pair with joint probabilities.
- 
- >Now let’s have Monty open door C again and see how that affects the probabilities.
- 
- >### The Hundred Doors Solution to The Monty Hall Problem.
- >
- >In this hypothetical version of the problem the contestant chooses not from 3 doors but from a hundred, for convenience we’ll label these doors 1–100 and say our contestant selects door 1. She has a 1/100 of selecting the door hiding the car and a 99/100 chance of not selecting a car. Let’s represent that with a stripped-down diagram. Again, black denotes the chance of selecting the car. Red the chance of not selecting the car.
- 
- >Now alternative universe Monty works his way through doors 2–100 careful revealing bearded goats until the bored contestant is left with two doors. Door 1 which she initially chose and door 2 the last remaining unchosen door. Here’s how the odds stack up at this point.
- 
#5: Post edited
- The second quotation below uses the verb "shift" to describe how Monty Hall's opening the 98 unchosen doors (revealing a goat each) ""shifts" [boldening mine] that 99/100 chance to door #100"? The first quotation below uses "transfer". But how can probabilities "shift" or "transfer"?
- This analogy sounds featherbrained, but I hope it explains my bafflement. Imagine a doorman beside each door. Each time after the host opens an unchosen door (revealing a goat), each doorman walks over and congregates at another random unchosen door. After the host opens $n - 2$ unpicked doors, then $n - 2$ doormen will have congregated at the lone unchosen door. But probabilities aren't like doormen! From the $n - 2$ unchosen doors, they can't then "relocate" or "shift" to the lone unchosen door!
1. Judea Pearl, The Book of Why (2018). Page 181 of 402.- >
- > As you can see from Figure 6.1, Door Opened is a collider. Once we
- obtain information on this variable, all our probabilities become conditional
- on this information. But when we condition on a collider, we create a spurious
- dependence between its parents. The dependence is borne out in the
- probabilities: if you chose Door 1, the car location is twice as likely to be
- behind Door 2 as Door 1; if you chose Door 2, the car location is twice as
- likely to be behind Door 1.
- > It is a bizarre dependence for sure, one of a type that most of us are
- unaccustomed to. It is a dependence that has no cause. It does not involve
- physical communication between the producers and us. It does not involve
- mental telepathy. It is purely an artifact of Bayesian conditioning: a magical
- transfer of information without causality. Our minds rebel at this possibility
- because from earliest infancy, we have learned to associate correlation with
- causation. If a car behind us takes all the same turns that we do, we first think
- it is following us (causation!). We next think that we are going to the same
- place (i.e., there is a common cause behind each of our turns). But causeless
- correlation violates our common sense. Thus, the Monty Hall paradox is just
- like an optical illusion or a magic trick: it uses our own cognitive machinery
- to deceive us.
- > Why do I say that Monty Hall’s opening of Door 3 was a “transfer of
- information”? It didn’t, after all, provide any evidence about whether your
- initial choice of Door 1 was correct. You knew in advance that he was going
- to open a door that hid a goat, and so he did. No one should ask you to change
- your beliefs if you witness the inevitable. So how come your belief in Door 2
- has gone up from one-third to two-thirds?
- 2. [The Time Everyone “Corrected” the World’s Smartest Woman](https://priceonomics.com/the-time-everyone-corrected-the-worlds-smartest/)
- > Here’s another way to visualize this. Imagine that instead of three doors, Monty Hall presents you with 100 doors; behind 99 of them are goats, and behind one of them is the car. You select door #1, and your initial odds of winning the car are now 1/100:
- >
- > 
- >
- > Then, let’s suppose that Monty Hall opens 98 of the other doors, revealing a goat behind each one. Now you’re left with two choices: keep door #1, or switch to door #100:
- >
- > 
- >
- > When you select door #1, there is a 99/100 chance that the car is behind one of the other doors. The fact that Monty Hall reveals 98 goats does not change these initial odds -- it merely **"shifts" [boldening mine]** that 99/100 chance to door #100. You can either stick with your original 1/100 odds pick, or switch to door #100, with a much higher probability of winning the car.
- 3. [by Robert Lea | Medium](https://medium.com/@roblea_63049/exploring-one-of-mathematics-most-enigmatic-puzzles-the-monty-hall-problem-f7b0beb8e1f1)
- 
- > At this point the switch shouldn’t seem attractive, there’s no benefit to changing. That all changes when Monty opens door C. This action means that there is then still a 2/3 chance that the car isn’t behind door A. This means there is a 2/3 chance the car is behind B or C. The opening of door C hasn’t affected the probabilities associated with door A but it has affected those associated with door B. It’s almost as if door B **must carry the full load [boldening mine]** of probabilities that was previously held by both B and C. This is because C isn’t chosen randomly. There is a 2/3 chance the car is behind the B&C pairing, that burden is left to B. Let’s look at that diagrammatically representing B & C as a pair with joint probabilities.
- 
- >Now let’s have Monty open door C again and see how that affects the probabilities.
- 
- >### The Hundred Doors Solution to The Monty Hall Problem.
- >
- >In this hypothetical version of the problem the contestant chooses not from 3 doors but from a hundred, for convenience we’ll label these doors 1–100 and say our contestant selects door 1. She has a 1/100 of selecting the door hiding the car and a 99/100 chance of not selecting a car. Let’s represent that with a stripped-down diagram. Again, black denotes the chance of selecting the car. Red the chance of not selecting the car.
- 
- >Now alternative universe Monty works his way through doors 2–100 careful revealing bearded goats until the bored contestant is left with two doors. Door 1 which she initially chose and door 2 the last remaining unchosen door. Here’s how the odds stack up at this point.
- 
- The second quotation below uses the verb "shift" to describe how Monty Hall's opening the 98 unchosen doors (revealing a goat each) ""shifts" [boldening mine] that 99/100 chance to door #100"? The first quotation below uses "transfer". But how can probabilities "shift" or "transfer"?
- This analogy sounds featherbrained, but I hope it explains my bafflement. Imagine a doorman beside each door. Each time after the host opens an unchosen door (revealing a goat), each doorman walks over and congregates at another random unchosen door. After the host opens $n - 2$ unpicked doors, then $n - 2$ doormen will have congregated at the lone unchosen door. But probabilities aren't like doormen! From the $n - 2$ unchosen doors, they can't then "relocate" or "shift" to the lone unchosen door!
- 1. Judea Pearl, _The Book of Why _(2018). Page 181 of 402.
- >
- > As you can see from Figure 6.1, Door Opened is a collider. Once we
- obtain information on this variable, all our probabilities become conditional
- on this information. But when we condition on a collider, we create a spurious
- dependence between its parents. The dependence is borne out in the
- probabilities: if you chose Door 1, the car location is twice as likely to be
- behind Door 2 as Door 1; if you chose Door 2, the car location is twice as
- likely to be behind Door 1.
- > It is a bizarre dependence for sure, one of a type that most of us are
- unaccustomed to. It is a dependence that has no cause. It does not involve
- physical communication between the producers and us. It does not involve
- mental telepathy. It is purely an artifact of Bayesian conditioning: a magical
- transfer of information without causality. Our minds rebel at this possibility
- because from earliest infancy, we have learned to associate correlation with
- causation. If a car behind us takes all the same turns that we do, we first think
- it is following us (causation!). We next think that we are going to the same
- place (i.e., there is a common cause behind each of our turns). But causeless
- correlation violates our common sense. Thus, the Monty Hall paradox is just
- like an optical illusion or a magic trick: it uses our own cognitive machinery
- to deceive us.
- > Why do I say that Monty Hall’s opening of Door 3 was a “transfer of
- information”? It didn’t, after all, provide any evidence about whether your
- initial choice of Door 1 was correct. You knew in advance that he was going
- to open a door that hid a goat, and so he did. No one should ask you to change
- your beliefs if you witness the inevitable. So how come your belief in Door 2
- has gone up from one-third to two-thirds?
- 2. [The Time Everyone “Corrected” the World’s Smartest Woman](https://priceonomics.com/the-time-everyone-corrected-the-worlds-smartest/)
- > Here’s another way to visualize this. Imagine that instead of three doors, Monty Hall presents you with 100 doors; behind 99 of them are goats, and behind one of them is the car. You select door #1, and your initial odds of winning the car are now 1/100:
- >
- > 
- >
- > Then, let’s suppose that Monty Hall opens 98 of the other doors, revealing a goat behind each one. Now you’re left with two choices: keep door #1, or switch to door #100:
- >
- > 
- >
- > When you select door #1, there is a 99/100 chance that the car is behind one of the other doors. The fact that Monty Hall reveals 98 goats does not change these initial odds -- it merely **"shifts" [boldening mine]** that 99/100 chance to door #100. You can either stick with your original 1/100 odds pick, or switch to door #100, with a much higher probability of winning the car.
- 3. [by Robert Lea | Medium](https://medium.com/@roblea_63049/exploring-one-of-mathematics-most-enigmatic-puzzles-the-monty-hall-problem-f7b0beb8e1f1)
- 
- > At this point the switch shouldn’t seem attractive, there’s no benefit to changing. That all changes when Monty opens door C. This action means that there is then still a 2/3 chance that the car isn’t behind door A. This means there is a 2/3 chance the car is behind B or C. The opening of door C hasn’t affected the probabilities associated with door A but it has affected those associated with door B. It’s almost as if door B **must carry the full load [boldening mine]** of probabilities that was previously held by both B and C. This is because C isn’t chosen randomly. There is a 2/3 chance the car is behind the B&C pairing, that burden is left to B. Let’s look at that diagrammatically representing B & C as a pair with joint probabilities.
- 
- >Now let’s have Monty open door C again and see how that affects the probabilities.
- 
- >### The Hundred Doors Solution to The Monty Hall Problem.
- >
- >In this hypothetical version of the problem the contestant chooses not from 3 doors but from a hundred, for convenience we’ll label these doors 1–100 and say our contestant selects door 1. She has a 1/100 of selecting the door hiding the car and a 99/100 chance of not selecting a car. Let’s represent that with a stripped-down diagram. Again, black denotes the chance of selecting the car. Red the chance of not selecting the car.
- 
- >Now alternative universe Monty works his way through doors 2–100 careful revealing bearded goats until the bored contestant is left with two doors. Door 1 which she initially chose and door 2 the last remaining unchosen door. Here’s how the odds stack up at this point.
- 
#4: Post edited
After $n - 2$ unchosen doors are opened, how does the probability of the $n - 2$ unchosen doors "shift" to the lone unchosen door?
- After $n - 2$ unchosen doors are opened, how does the probability of the $n - 2$ unchosen doors "shift" or "transfer" to the lone unchosen door?
#3: Post edited
The first quotation below uses the verb "shift" to describe how Monty Hall's opening the 98 unchosen doors (revealing a goat each) ""shifts" [boldening mine] that 99/100 chance to door #100"? But how can probabilities "shift" like this"?- This analogy sounds featherbrained, but I hope it explains my bafflement. Imagine a doorman beside each door. Each time after the host opens an unchosen door (revealing a goat), each doorman walks over and congregates at another random unchosen door. After the host opens $n - 2$ unpicked doors, then $n - 2$ doormen will have congregated at the lone unchosen door. But probabilities aren't like doormen! From the $n - 2$ unchosen doors, they can't then "relocate" or "shift" to the lone unchosen door!
[The Time Everyone “Corrected” the World’s Smartest Woman](https://priceonomics.com/the-time-everyone-corrected-the-worlds-smartest/)- > Here’s another way to visualize this. Imagine that instead of three doors, Monty Hall presents you with 100 doors; behind 99 of them are goats, and behind one of them is the car. You select door #1, and your initial odds of winning the car are now 1/100:
- >
- > 
- >
- > Then, let’s suppose that Monty Hall opens 98 of the other doors, revealing a goat behind each one. Now you’re left with two choices: keep door #1, or switch to door #100:
- >
- > 
- >
- > When you select door #1, there is a 99/100 chance that the car is behind one of the other doors. The fact that Monty Hall reveals 98 goats does not change these initial odds -- it merely **"shifts" [boldening mine]** that 99/100 chance to door #100. You can either stick with your original 1/100 odds pick, or switch to door #100, with a much higher probability of winning the car.
[by Robert Lea | Medium](https://medium.com/@roblea_63049/exploring-one-of-mathematics-most-enigmatic-puzzles-the-monty-hall-problem-f7b0beb8e1f1)- 
- > At this point the switch shouldn’t seem attractive, there’s no benefit to changing. That all changes when Monty opens door C. This action means that there is then still a 2/3 chance that the car isn’t behind door A. This means there is a 2/3 chance the car is behind B or C. The opening of door C hasn’t affected the probabilities associated with door A but it has affected those associated with door B. It’s almost as if door B **must carry the full load [boldening mine]** of probabilities that was previously held by both B and C. This is because C isn’t chosen randomly. There is a 2/3 chance the car is behind the B&C pairing, that burden is left to B. Let’s look at that diagrammatically representing B & C as a pair with joint probabilities.
- 
- >Now let’s have Monty open door C again and see how that affects the probabilities.
- 
- >### The Hundred Doors Solution to The Monty Hall Problem.
- >
- >In this hypothetical version of the problem the contestant chooses not from 3 doors but from a hundred, for convenience we’ll label these doors 1–100 and say our contestant selects door 1. She has a 1/100 of selecting the door hiding the car and a 99/100 chance of not selecting a car. Let’s represent that with a stripped-down diagram. Again, black denotes the chance of selecting the car. Red the chance of not selecting the car.
- 
- >Now alternative universe Monty works his way through doors 2–100 careful revealing bearded goats until the bored contestant is left with two doors. Door 1 which she initially chose and door 2 the last remaining unchosen door. Here’s how the odds stack up at this point.
- 
- The second quotation below uses the verb "shift" to describe how Monty Hall's opening the 98 unchosen doors (revealing a goat each) ""shifts" [boldening mine] that 99/100 chance to door #100"? The first quotation below uses "transfer". But how can probabilities "shift" or "transfer"?
- This analogy sounds featherbrained, but I hope it explains my bafflement. Imagine a doorman beside each door. Each time after the host opens an unchosen door (revealing a goat), each doorman walks over and congregates at another random unchosen door. After the host opens $n - 2$ unpicked doors, then $n - 2$ doormen will have congregated at the lone unchosen door. But probabilities aren't like doormen! From the $n - 2$ unchosen doors, they can't then "relocate" or "shift" to the lone unchosen door!
- 1. Judea Pearl, The Book of Why (2018). Page 181 of 402.
- >
- > As you can see from Figure 6.1, Door Opened is a collider. Once we
- obtain information on this variable, all our probabilities become conditional
- on this information. But when we condition on a collider, we create a spurious
- dependence between its parents. The dependence is borne out in the
- probabilities: if you chose Door 1, the car location is twice as likely to be
- behind Door 2 as Door 1; if you chose Door 2, the car location is twice as
- likely to be behind Door 1.
- > It is a bizarre dependence for sure, one of a type that most of us are
- unaccustomed to. It is a dependence that has no cause. It does not involve
- physical communication between the producers and us. It does not involve
- mental telepathy. It is purely an artifact of Bayesian conditioning: a magical
- transfer of information without causality. Our minds rebel at this possibility
- because from earliest infancy, we have learned to associate correlation with
- causation. If a car behind us takes all the same turns that we do, we first think
- it is following us (causation!). We next think that we are going to the same
- place (i.e., there is a common cause behind each of our turns). But causeless
- correlation violates our common sense. Thus, the Monty Hall paradox is just
- like an optical illusion or a magic trick: it uses our own cognitive machinery
- to deceive us.
- > Why do I say that Monty Hall’s opening of Door 3 was a “transfer of
- information”? It didn’t, after all, provide any evidence about whether your
- initial choice of Door 1 was correct. You knew in advance that he was going
- to open a door that hid a goat, and so he did. No one should ask you to change
- your beliefs if you witness the inevitable. So how come your belief in Door 2
- has gone up from one-third to two-thirds?
- 2. [The Time Everyone “Corrected” the World’s Smartest Woman](https://priceonomics.com/the-time-everyone-corrected-the-worlds-smartest/)
- > Here’s another way to visualize this. Imagine that instead of three doors, Monty Hall presents you with 100 doors; behind 99 of them are goats, and behind one of them is the car. You select door #1, and your initial odds of winning the car are now 1/100:
- >
- > 
- >
- > Then, let’s suppose that Monty Hall opens 98 of the other doors, revealing a goat behind each one. Now you’re left with two choices: keep door #1, or switch to door #100:
- >
- > 
- >
- > When you select door #1, there is a 99/100 chance that the car is behind one of the other doors. The fact that Monty Hall reveals 98 goats does not change these initial odds -- it merely **"shifts" [boldening mine]** that 99/100 chance to door #100. You can either stick with your original 1/100 odds pick, or switch to door #100, with a much higher probability of winning the car.
- 3. [by Robert Lea | Medium](https://medium.com/@roblea_63049/exploring-one-of-mathematics-most-enigmatic-puzzles-the-monty-hall-problem-f7b0beb8e1f1)
- 
- > At this point the switch shouldn’t seem attractive, there’s no benefit to changing. That all changes when Monty opens door C. This action means that there is then still a 2/3 chance that the car isn’t behind door A. This means there is a 2/3 chance the car is behind B or C. The opening of door C hasn’t affected the probabilities associated with door A but it has affected those associated with door B. It’s almost as if door B **must carry the full load [boldening mine]** of probabilities that was previously held by both B and C. This is because C isn’t chosen randomly. There is a 2/3 chance the car is behind the B&C pairing, that burden is left to B. Let’s look at that diagrammatically representing B & C as a pair with joint probabilities.
- 
- >Now let’s have Monty open door C again and see how that affects the probabilities.
- 
- >### The Hundred Doors Solution to The Monty Hall Problem.
- >
- >In this hypothetical version of the problem the contestant chooses not from 3 doors but from a hundred, for convenience we’ll label these doors 1–100 and say our contestant selects door 1. She has a 1/100 of selecting the door hiding the car and a 99/100 chance of not selecting a car. Let’s represent that with a stripped-down diagram. Again, black denotes the chance of selecting the car. Red the chance of not selecting the car.
- 
- >Now alternative universe Monty works his way through doors 2–100 careful revealing bearded goats until the bored contestant is left with two doors. Door 1 which she initially chose and door 2 the last remaining unchosen door. Here’s how the odds stack up at this point.
- 
#2: Post edited
After $n - 2$ doors are opened, how does the probability of the $n - 2$ doors "shift" to the lone unopened door?
- After $n - 2$ unchosen doors are opened, how does the probability of the $n - 2$ unchosen doors "shift" to the lone unchosen door?
- [The Time Everyone “Corrected” the World’s Smartest Woman](https://priceonomics.com/the-time-everyone-corrected-the-worlds-smartest/)
- > Here’s another way to visualize this. Imagine that instead of three doors, Monty Hall presents you with 100 doors; behind 99 of them are goats, and behind one of them is the car. You select door #1, and your initial odds of winning the car are now 1/100:
- >
- > 
- >
- > Then, let’s suppose that Monty Hall opens 98 of the other doors, revealing a goat behind each one. Now you’re left with two choices: keep door #1, or switch to door #100:
- >
- > 
- >
- > When you select door #1, there is a 99/100 chance that the car is behind one of the other doors. The fact that Monty Hall reveals 98 goats does not change these initial odds -- it merely **"shifts" [boldening mine]** that 99/100 chance to door #100. You can either stick with your original 1/100 odds pick, or switch to door #100, with a much higher probability of winning the car.
- [by Robert Lea | Medium](https://medium.com/@roblea_63049/exploring-one-of-mathematics-most-enigmatic-puzzles-the-monty-hall-problem-f7b0beb8e1f1)
- 
- > At this point the switch shouldn’t seem attractive, there’s no benefit to changing. That all changes when Monty opens door C. This action means that there is then still a 2/3 chance that the car isn’t behind door A. This means there is a 2/3 chance the car is behind B or C. The opening of door C hasn’t affected the probabilities associated with door A but it has affected those associated with door B. It’s almost as if door B **must carry the full load [boldening mine]** of probabilities that was previously held by both B and C. This is because C isn’t chosen randomly. There is a 2/3 chance the car is behind the B&C pairing, that burden is left to B. Let’s look at that diagrammatically representing B & C as a pair with joint probabilities.
- 
- >Now let’s have Monty open door C again and see how that affects the probabilities.
- 
- >### The Hundred Doors Solution to The Monty Hall Problem.
- >
- >In this hypothetical version of the problem the contestant chooses not from 3 doors but from a hundred, for convenience we’ll label these doors 1–100 and say our contestant selects door 1. She has a 1/100 of selecting the door hiding the car and a 99/100 chance of not selecting a car. Let’s represent that with a stripped-down diagram. Again, black denotes the chance of selecting the car. Red the chance of not selecting the car.
- 
- >Now alternative universe Monty works his way through doors 2–100 careful revealing bearded goats until the bored contestant is left with two doors. Door 1 which she initially chose and door 2 the last remaining unchosen door. Here’s how the odds stack up at this point.
- 
- The first quotation below uses the verb "shift" to describe how Monty Hall's opening the 98 unchosen doors (revealing a goat each) ""shifts" [boldening mine] that 99/100 chance to door #100"? But how can probabilities "shift" like this"?
- This analogy sounds featherbrained, but I hope it explains my bafflement. Imagine a doorman beside each door. Each time after the host opens an unchosen door (revealing a goat), each doorman walks over and congregates at another random unchosen door. After the host opens $n - 2$ unpicked doors, then $n - 2$ doormen will have congregated at the lone unchosen door. But probabilities aren't like doormen! From the $n - 2$ unchosen doors, they can't then "relocate" or "shift" to the lone unchosen door!
- [The Time Everyone “Corrected” the World’s Smartest Woman](https://priceonomics.com/the-time-everyone-corrected-the-worlds-smartest/)
- > Here’s another way to visualize this. Imagine that instead of three doors, Monty Hall presents you with 100 doors; behind 99 of them are goats, and behind one of them is the car. You select door #1, and your initial odds of winning the car are now 1/100:
- >
- > 
- >
- > Then, let’s suppose that Monty Hall opens 98 of the other doors, revealing a goat behind each one. Now you’re left with two choices: keep door #1, or switch to door #100:
- >
- > 
- >
- > When you select door #1, there is a 99/100 chance that the car is behind one of the other doors. The fact that Monty Hall reveals 98 goats does not change these initial odds -- it merely **"shifts" [boldening mine]** that 99/100 chance to door #100. You can either stick with your original 1/100 odds pick, or switch to door #100, with a much higher probability of winning the car.
- [by Robert Lea | Medium](https://medium.com/@roblea_63049/exploring-one-of-mathematics-most-enigmatic-puzzles-the-monty-hall-problem-f7b0beb8e1f1)
- 
- > At this point the switch shouldn’t seem attractive, there’s no benefit to changing. That all changes when Monty opens door C. This action means that there is then still a 2/3 chance that the car isn’t behind door A. This means there is a 2/3 chance the car is behind B or C. The opening of door C hasn’t affected the probabilities associated with door A but it has affected those associated with door B. It’s almost as if door B **must carry the full load [boldening mine]** of probabilities that was previously held by both B and C. This is because C isn’t chosen randomly. There is a 2/3 chance the car is behind the B&C pairing, that burden is left to B. Let’s look at that diagrammatically representing B & C as a pair with joint probabilities.
- 
- >Now let’s have Monty open door C again and see how that affects the probabilities.
- 
- >### The Hundred Doors Solution to The Monty Hall Problem.
- >
- >In this hypothetical version of the problem the contestant chooses not from 3 doors but from a hundred, for convenience we’ll label these doors 1–100 and say our contestant selects door 1. She has a 1/100 of selecting the door hiding the car and a 99/100 chance of not selecting a car. Let’s represent that with a stripped-down diagram. Again, black denotes the chance of selecting the car. Red the chance of not selecting the car.
- 
- >Now alternative universe Monty works his way through doors 2–100 careful revealing bearded goats until the bored contestant is left with two doors. Door 1 which she initially chose and door 2 the last remaining unchosen door. Here’s how the odds stack up at this point.
- 
#1: Initial revision
After $n - 2$ doors are opened, how does the probability of the $n - 2$ doors "shift" to the lone unopened door?
[The Time Everyone “Corrected” the World’s Smartest Woman](https://priceonomics.com/the-time-everyone-corrected-the-worlds-smartest/) > Here’s another way to visualize this. Imagine that instead of three doors, Monty Hall presents you with 100 doors; behind 99 of them are goats, and behind one of them is the car. You select door #1, and your initial odds of winning the car are now 1/100: > >  > > Then, let’s suppose that Monty Hall opens 98 of the other doors, revealing a goat behind each one. Now you’re left with two choices: keep door #1, or switch to door #100: > >  > > When you select door #1, there is a 99/100 chance that the car is behind one of the other doors. The fact that Monty Hall reveals 98 goats does not change these initial odds -- it merely **"shifts" [boldening mine]** that 99/100 chance to door #100. You can either stick with your original 1/100 odds pick, or switch to door #100, with a much higher probability of winning the car. [by Robert Lea | Medium](https://medium.com/@roblea_63049/exploring-one-of-mathematics-most-enigmatic-puzzles-the-monty-hall-problem-f7b0beb8e1f1)  > At this point the switch shouldn’t seem attractive, there’s no benefit to changing. That all changes when Monty opens door C. This action means that there is then still a 2/3 chance that the car isn’t behind door A. This means there is a 2/3 chance the car is behind B or C. The opening of door C hasn’t affected the probabilities associated with door A but it has affected those associated with door B. It’s almost as if door B **must carry the full load [boldening mine]** of probabilities that was previously held by both B and C. This is because C isn’t chosen randomly. There is a 2/3 chance the car is behind the B&C pairing, that burden is left to B. Let’s look at that diagrammatically representing B & C as a pair with joint probabilities.  >Now let’s have Monty open door C again and see how that affects the probabilities.  >### The Hundred Doors Solution to The Monty Hall Problem. > >In this hypothetical version of the problem the contestant chooses not from 3 doors but from a hundred, for convenience we’ll label these doors 1–100 and say our contestant selects door 1. She has a 1/100 of selecting the door hiding the car and a 99/100 chance of not selecting a car. Let’s represent that with a stripped-down diagram. Again, black denotes the chance of selecting the car. Red the chance of not selecting the car.  >Now alternative universe Monty works his way through doors 2–100 careful revealing bearded goats until the bored contestant is left with two doors. Door 1 which she initially chose and door 2 the last remaining unchosen door. Here’s how the odds stack up at this point. 


















