Post History

How does $\lim\limits_{n \to \infty} \dfrac{p[s + (1 - s)c^n]}{p[ s + (1 - s)c^n] + (1 - p)[s + (1 - s)w^n]} = p?$

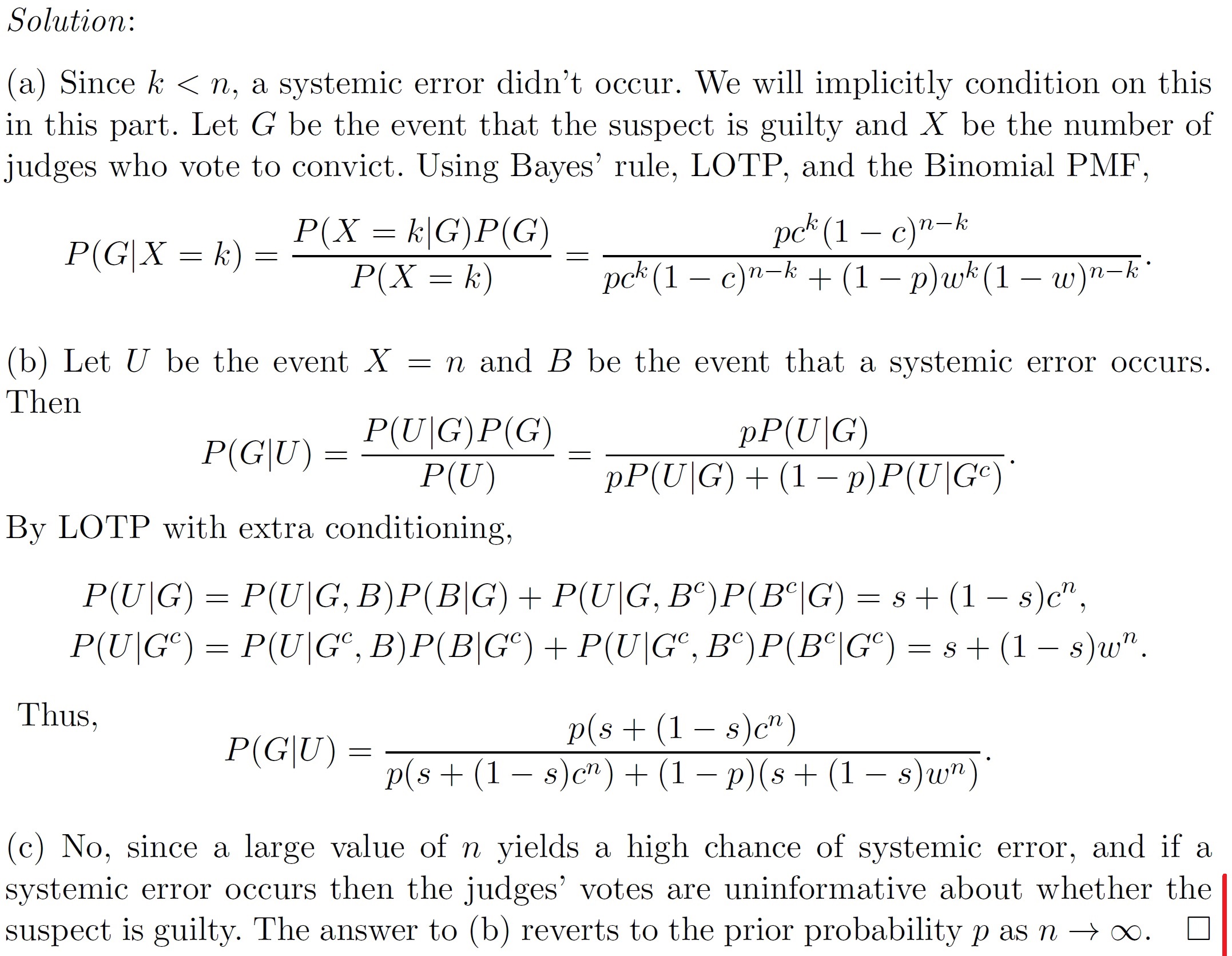
To minimize this post's length, I don't repeat the [exercise itself](https://math.codidact.com/posts/282889). The title refers to the sentence beside my red line below. 

I divide both the numerator and denominator by $\color{red}{c^n}$. Then $P(G|U) = \dfrac{p[
\dfrac{s}{\color{red}{c^n}} + (1 - s)]}{p[
\dfrac{s}{\color{red}{c^n}} + (1 - s)] + \dfrac{s(1 - p)}{\color{red}{c^n}} + (1-p)(1-s)\dfrac{w^n}{\color{red}{c^n}}} $.

The question postulates $0 < w < 0.5 < c < 1$, but this $\iff 0 < \dfrac{w^n}{c^n} < \dfrac{0.5^n}{c^n} < 1 < \dfrac{1}{c^n} $. So if $n \to \infty$, then all terms above containing ${\color{red}{c^n}} \to 0$. 

Then $\lim\limits_{n \to \infty} P(G|U) =\dfrac{p(1 - s)}{p(1 - s)} = 1 \neq p$. What did I flub? 

![Image alt text](https://math.codidact.com/uploads/UwffXacuhHdz1Aogv8uT1suL)


Blitzstein, *Introduction to Probability* (2019 2 ed), Example 2.4.5, p 62.