Post History

Why isn't the probability of being void in 3 specific suits $\frac{1/13}{\dbinom{52}{13}}$?

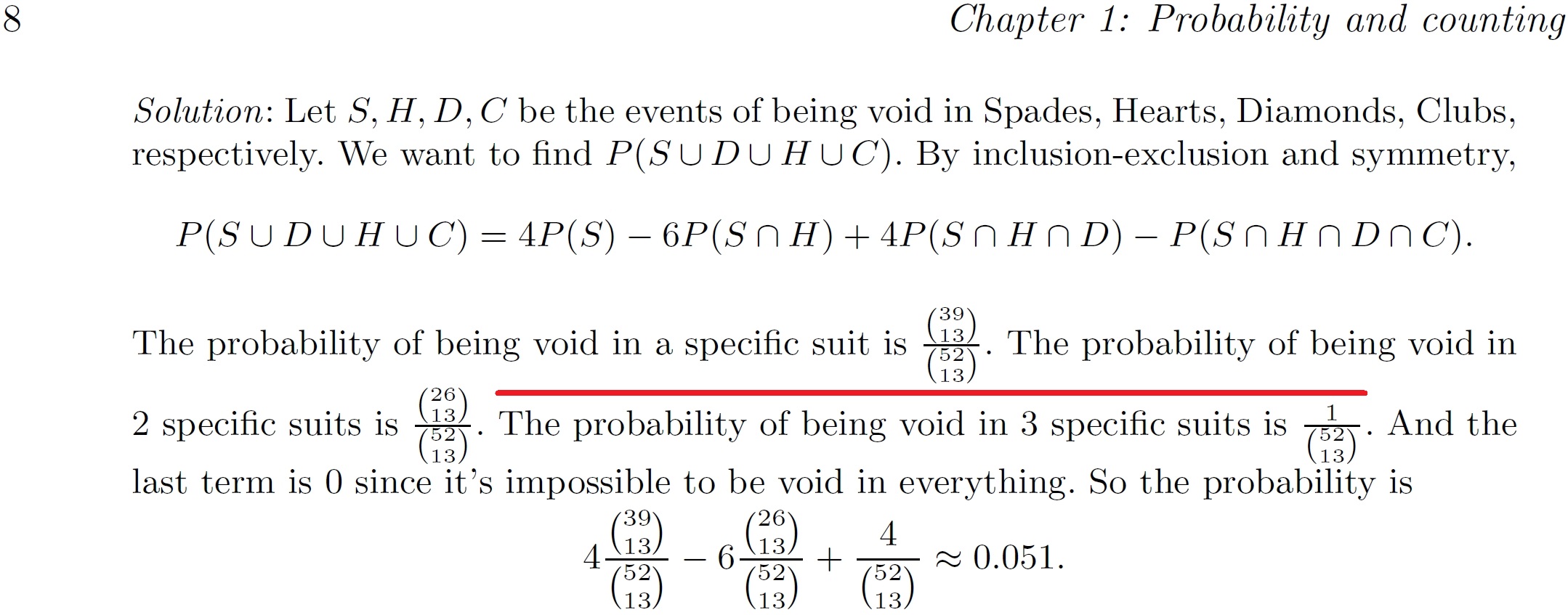
Kindly see the sentence UNDER the red line below. Why isn't the probability being void in 3 specific suits $\frac{1/13}{\dbinom{52}{13}}$? As "the probability of being void in 3 specific suits" means you don't want cards of 3 suits, you desire cards of solely ONE suit. If you remove all cards of any 3 suits, then just 13 cards (of the same suit) remain. Thus the probability that you pick a card of solely one suit is $1/13$.

>50. A card player is dealt a 13-card hand from a well-shuffled, standard deck of cards.
What is the probability that the hand is void in at least one suit ("void in a suit" means
having no cards of that suit)?

>![Image alt text](https://math.codidact.com/uploads/ztd6fQ2xpjdGxwymYmEU6u83)

Blitzstein, *Introduction to Probability* (2019 2 ed), p 40, Exercise 50. Selected Solutions PDF, p 7.