Post History
#3: Post edited
by
Wolgwang
·
2021-09-02T14:14:35Z (over 3 years ago)
Inserted text and MathJax from the images for better look and search efficiency
Without calculations, how can you visualize "that half the squares are white and half are black"?
Please see the 2nd para. below alongside my red line. I can't "[i]magine rotating the chessboard 90 degrees clockwise." I can't visualize how "all the positions that had a white square now contain a black square, and vice- versa".
- Blitzstein. *Introduction to Probability* (2019 2 ed). Pages 9-10.
- Please see the 2nd para. below alongside my red highlighted words. I can't "[i]magine rotating the chessboard 90 degrees clockwise." I can't visualize how "all the positions that had a white square now contain a black square, and vice
- versa".
- <hr>
- Example 1.4.4 (Chessboard). How many squares are there in an $8 \times 8$ chessboard, as in Figure $1.3$ ? Even the name " $8 \times 8$ chessboard" makes this easy: there are $8 \cdot 8=64$ squares on the board. The grid structure makes this clear, but we can also think of this as an example of the multiplication rule: to specify a square, we can specify which row and which column it is in. There are 8 choices of row, for each of which there are 8 choices of column.
- Furthermore, we can see without doing any calculations that $\color{red}{\text{half the squares are white and half are black}}$. Imagine rotating the chessboard 90 degrees clockwise. Then all the positions that had a white square now contain a black square, and vice versa, so the number of white squares must equal the number of black squares.
- 
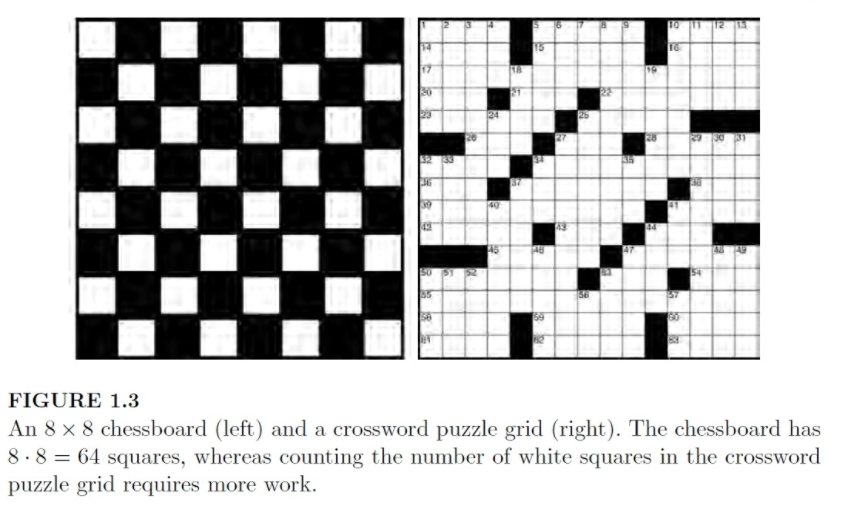
- We can also count the number of white squares using the multiplication rule: in each of the 8 rows there are 4 white squares, giving a total of $8 \cdot 4=32$ white squares.
- In contrast, it would require more effort to count the number of white squares in the crossword puzzle grid shown in Figure 1.3. The multiplication rule does not apply, since different rows sometimes have different numbers of white squares.
- [Original image](https://i.imgur.com/2OOMCNF.jpg)
- Blitzstein. *Introduction to Probability* (2019 2 ed). Pages 9-10.
#2: Post edited
by
DNB
·
2021-07-09T03:52:38Z (over 3 years ago)
- Please see the 2nd para. below alongside my red line. I can't "[i]magine rotating the chessboard 90 degrees clockwise." I can't visualize how "all the positions that had a white square now contain a black square, and vice
- versa".
- 
Blitzstein, Hwang. *Introduction to Probability* (2019 2 ed). Pages 9-10.
- Please see the 2nd para. below alongside my red line. I can't "[i]magine rotating the chessboard 90 degrees clockwise." I can't visualize how "all the positions that had a white square now contain a black square, and vice
- versa".
- 
- Blitzstein. *Introduction to Probability* (2019 2 ed). Pages 9-10.
#1: Initial revision
by
DNB
·
2021-07-09T03:50:39Z (over 3 years ago)
Without calculations, how can you visualize "that half the squares are white and half are black"?
Please see the 2nd para. below alongside my red line. I can't "[i]magine rotating the chessboard 90 degrees clockwise." I can't visualize how "all the positions that had a white square now contain a black square, and vice versa".  Blitzstein, Hwang. *Introduction to Probability* (2019 2 ed). Pages 9-10.